Back to Online Manuals

7965x

002715

2023-12-15

Select Manual

Overview

Graphical User Interface and Settings

Dlubal Center

Model Base Data

Structure

Imperfections

Load Cases and Combinations

Load Wizards

Loads

Calculation

Results

Guide Objects

Program Functions

Result Evaluation

Printout Reports

Stresses

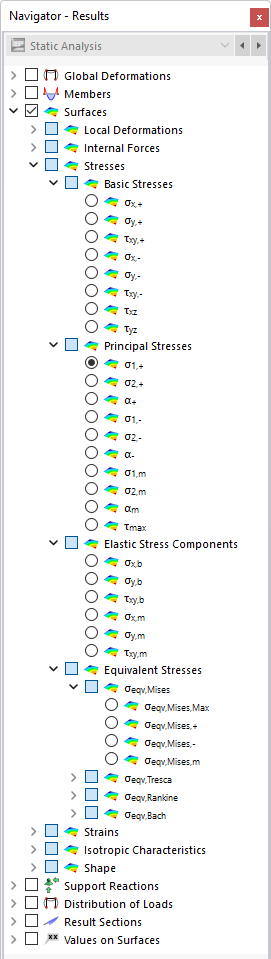
In the navigator, define the stresses to be displayed on the surfaces. The table lists the stresses of each surface according to the specifications set in the Result Table Manager .

Selecting Surface Stresses in Navigator

Surface Stresses in Table

The surface stresses are subdivided into the following categories:

Basic Stresses: stresses in the direction of the surface axes
Principal Stresses: stresses in the direction of the principal axes
Elastic Stress Components: stresses from moments and axial forces
Equivalent Stresses: stresses according to various equivalent stress hypotheses

Basic stresses

Basic stresses are related to the directions of the local surface axes. When analyzing curved surfaces, they refer to the local axes of finite elements (see image Displaying FE Axis Systems ).

The basic stresses are shown in the Surface Internal Forces and Surface Stresses image. They have the following meanings:

σ_{x, +} = \frac{n_{x}}{d} + \frac{6 m_{x}}{d^{2}}

d	thickness of surface

Stress σ_x,+ (in Direction of Local x-Axis on Positive Surface Side)

σ_{y, +} = \frac{n_{y}}{d} + \frac{6 m_{y}}{d^{2}}

Stress σ_y,+ (in Direction of Local y-Axis on Positive Surface Side)

σ_{x, -} = \frac{n_{x}}{d} - \frac{6 m_{x}}{d^{2}}

Stress σ_x,- (in Direction of x-Axis on Negative Surface Side)

σ_{y, -} = \frac{n_{y}}{d} - \frac{6 m_{y}}{d^{2}}

Stress σ_y,- (in Direction of y-Axis on Negative Surface Side)

τ_{x y, +} = \frac{n_{x y}}{d} + \frac{6 m_{x y}}{d^{2}}

Torsional Stress τ_xy,+ on Positive Surface Side

τ_{x y, -} = \frac{n_{x y}}{d} - \frac{6 m_{x y}}{d^{2}}

Torsional Stress τ_xy,- on Negative Surface Side

τ_{x z} = \frac{3 v_{x}}{2 d}

Shear Stress τ_xz Perpendicular to Surface in Direction of x-Axis

τ_{y z} = \frac{3 v_{y}}{2 d}

Shear Stress τ_yz Perpendicular to Durface in Direction of y-Axis

Principal stresses

While the basic stresses refer to the xyz coordinate system of a surface, the principal stresses represent the extreme values of the stresses in a surface element. The principal axes 1 (maximum value) and 2 (minimum value) are arranged orthogonally. It is possible to graphically display the principal axis directions α as Trajectories (compare image Displaying Trajectories of Principal Axes ).

The principal stresses are determined from the basic stresses as follows:

σ_{1, +} = \frac{1}{2} [σ_{x, +} + σ_{y, +} + \sqrt{{(σ_{x, +} - σ_{y, +})}^{2} + 4 {τ_{x y, +}}^{2}}]

Stress σ_1,+ in Direction of Principal Axis 1 on Positive Surface Side

σ_{2, +} = \frac{1}{2} [σ_{x, +} + σ_{y, +} - \sqrt{{(σ_{x, +} - σ_{y, +})}^{2} + 4 {τ_{x y, +}}^{2}}]

Stress σ_2,+ in Direction of Principal Axis 2 on Positive Surface Side

α_{+} = \frac{1}{2} \arctan 2 (\frac{2 τ_{x y, +}}{σ_{x, +} - σ_{y, +}}) \in (- 90 °, 90 °]

Angle α₊ Between Local Axis x (or y) and Principal Axis 1 (or 2) for Stresses on Positive Surface Side

σ_{1, -} = \frac{1}{2} [σ_{x, -} + σ_{y, -} + \sqrt{{(σ_{x, -} - σ_{y, -})}^{2} + 4 {τ_{x y, -}}^{2}}]

Stress σ_1,- in Direction of Principal Axis 1 on Negative Surface Side

σ_{2, -} = \frac{1}{2} [σ_{x, -} + σ_{y, -} - \sqrt{{(σ_{x, -} - σ_{y, -})}^{2} + 4 {τ_{x y, -}}^{2}}]

Stress σ_2,- in Direction of Principal Axis 2 on Negative Surface Side

α_{-} = \frac{1}{2} \arctan 2 (\frac{2 τ_{x y, -}}{σ_{x, -} - σ_{y, -}}) \in (- 90 °, 90 °]

Angle α_- Between Local Axis x (or y) and Principal Axis 1 (or 2) for Stresses on Negative Surface Side

σ_{1, m} = \frac{1}{2} [σ_{x, m} + σ_{y, m} + \sqrt{{(σ_{x, m} - σ_{y, m})}^{2} + 4 {τ_{x y, m}}^{2}}]

Membrane Stress σ_1,m in Direction of Principal Axis 1

σ_{2, m} = \frac{1}{2} [σ_{x, m} + σ_{y, m} - \sqrt{{(σ_{x, m} - σ_{y, m})}^{2} + 4 {τ_{x y, m}}^{2}}]

Membrane Stress σ_2,m in Direction of Principal Axis 2

α_{m} = \frac{1}{2} \arctan 2 (\frac{2 τ_{x y, m}}{σ_{x, m} - σ_{y, m}}) \in (- 90 °, 90 °]

Angle α_m Between Local Axis x and Principal Axis 1 for Membrane Stresses

τ_{\max} = \sqrt{{τ_{x z}}^{2} + {τ_{y z}}^{2}}

Maximum Shear Stress τ_max Perpendicular to Surface

Other stresses/Elastic stress components

This category includes stress components due to bending moments and membrane forces. They are related to the directions of the local surface axes. When curved surfaces are analyzed, they refer to the axes of the finite elements.

The bending and membrane stresses have the following meanings:

σ_{x, b} = \frac{6 m_{x}}{d^{2}}

d	Thickness of the surface

Stress σ_x,b Due to Bending Moment m_x

σ_{y, b} = \frac{6 m_{y}}{d^{2}}

Stress σ_y,b Due to Bending Moment m_y

τ_{x y, b} = \frac{6 m_{x y}}{d^{2}}

Stress τ_xy,b Due to Torsional Moment m_xy

σ_{x, m} = \frac{n_{x}}{d}

Membrane Stress σ_x,m Due to Axial Force n_x

σ_{y, m} = \frac{n_{y}}{d}

Membrane Stress σ_y,m Due to Axial Force n_y

τ_{x y, m} = \frac{n_{x y}}{d}

Stress τ_{xy, m} Due to Shear Flow n_xy

Equivalent stresses

The Basic Stresses are combined according to four equivalent stress hypotheses for the plane stress state.

von Mises

The approach by von Mises is also called "shape modification hypothesis". It is based on the assumption that the material fails when the distortion strain energy exceeds a certain limit. This energy is the type of energy that causes a distortion or deformation of the object. This approach represents the most well-known and most frequently used equivalent stress hypothesis. It is suitable for all materials that are not brittle. Therefore, it is widely used in steel building construction. The von Mises hypothesis is not suitable for hydrostatic stress conditions with equal principal stresses in all directions, as the equivalent stress is zero in such a case.

The equivalent stresses according to von Mises for the plane state of stress have the following meanings:

σ_{v, Mises, Max} = \max (σ_{v, Mises, +}; σ_{v, Mises, -})

Maximum Equivalent Stress σ_v,Mises,max on Positive or Negative Surface Side

σ_{v, Mises, +} = \sqrt{{σ_{x, +}}^{2} + {σ_{y, +}}^{2} - σ_{x, +} σ_{y, +} + 3 {τ_{x y, +}}^{2}}

Equivalent Stress σ_v,Mises,+ on Positive Surface Side

σ_{v, Mises, -} = \sqrt{{σ_{x, -}}^{2} + {σ_{y, -}}^{2} - σ_{x, -} σ_{y, -} + 3 {τ_{x y, -}}^{2}}

Equivalent Stress σ_v,Mises,- on Negative Surface Side

σ_{v, Mises, m} = \sqrt{{σ_{x, m}}^{2} + {σ_{y, m}}^{2} - σ_{x, m} σ_{y, m} + 3 {τ_{x y, m}}^{2}}

Membrane Equivalent Stress σ_v,Mises,m

Tresca

The approach by Tresca is also known as the "maximum shear stress theory". It is assumed that failure is caused by the maximum shear stress. As this hypothesis is especially applicable for brittle materials, it is frequently used in mechanical engineering.

The equivalent stresses according to Tresca are determined as follows:

σ_{v, Tresca, Max} = \max (σ_{v, Tresca, +}; σ_{v, Tresca, -})

Maximum Equivalent Stress σ_v,Tresca,max on Positive or Negative Surface Side

σ_{v, Tresca, +} = \max (|σ_{1, +} - σ_{2, +}|; |σ_{2, +}|; |σ_{1, +}|) or

σ_{v, Tresca, +} = \max (\sqrt{{(σ_{x, +} - σ_{y, +})}^{2} + 4 {τ_{xy, +}}^{2}}; |σ_{2, +}|; |σ_{1, +}|)

Equivalent Stress σ_v,Tresca,+ on Positive Surface Side

σ_{v, Tresca, -} = \max (|σ_{1, -} - σ_{2, -}|; |σ_{2, -}|; |σ_{1, -}|) or

σ_{v, Tresca, -} = \max (\sqrt{{(σ_{x, -} - σ_{y, -})}^{2} + 4 {τ_{xy, -}}^{2}}; |σ_{2, -}|; |σ_{1, -}|)

Equivalent Stress σ_v,Tresca,- on Negative Surface Side

σ_{v, Tresca, m} = \max (|σ_{1, m} - σ_{2, m}|; |σ_{2, m}|; |σ_{1, m}|) or

σ_{v, Tresca, m} = \max (\sqrt{{(σ_{x, m} - σ_{y, m})}^{2} + 4 {τ_{xy, m}}^{2}}; |σ_{2, m}|; |σ_{1, m}|)

Membrane Equivalent Stress σ_v,Tresca,m

Rankine

The equivalent stress hypothesis by Rankine is also known as the "maximum principal stress criterion". It is assumed that the maximum principal stress leads to failure.

The equivalent stresses according to Rankine are determined as follows:

σ_{v, Rankine, Max} = \max (σ_{v, Rankine, +}; σ_{v, Rankine, -})

Maximum Equivalent Stress σ_{v,Rankine,max} on Positive or Negative Surface Side

σ_{v, Rankine, +} = \frac{1}{2} |σ_{x, +} + σ_{y, +}| + \frac{1}{2} \sqrt{{(σ_{x, +} - σ_{y, +})}^{2} + 4 {τ_{xy, +}}^{2}}

Equivalent Stress σ_v,Rankine,+ on Positive Surface Side

σ_{v, Rankine, -} = \frac{1}{2} |σ_{x, -} + σ_{y, -}| + \frac{1}{2} \sqrt{{(σ_{x, -} - σ_{y, -})}^{2} + 4 {τ_{xy, -}}^{2}}

Equivalent Stress σ_v,Rankine,- on Negative Side of Surface

σ_{v, Rankine, m} = \frac{1}{2} |σ_{x, m} + σ_{y, m}| + \frac{1}{2} \sqrt{{(σ_{x, m} - σ_{y, m})}^{2} + 4 {τ_{xy, m}}^{2}}

Equivalent Stress σ_{v, Rankine,m}

Bach

The equivalent stress hypothesis according to Bach is also referred to as the "principal strain hypothesis". It is assumed that failure occurs in the direction of the greatest strain. This statement is similar to the stress analysis according to Rankine. However, the principal strain is used instead of the principal stress in this case.

The equivalent stresses according to Bach are determined as follows:

σ_{v, Bach, Max} = \max (σ_{v, Bach, +}; σ_{v, Bach, -})

Maximum Equivalent Stress σ_v,Bach,max on Positive or Negative Surface Side

σ_{v, Bach, +} = \max (\frac{1 - ν}{2} |σ_{x, +} + σ_{y, +}| + \frac{1 + ν}{2} \sqrt{{(σ_{x, +} - σ_{y, +})}^{2} + 4 {τ_{xy, +}}^{2}}; ν |σ_{x, +} + σ_{y, +}|)

ν	Poisson's ratio

Equivalent Stress σ_v,Bach,+ on Positive Surface Side

σ_{v, Bach, -} = \max (\frac{1 - ν}{2} |σ_{x, -} + σ_{y, -}| + \frac{1 + ν}{2} \sqrt{{(σ_{x, -} - σ_{y, -})}^{2} + 4 {τ_{xy, -}}^{2}}; ν |σ_{x, -} + σ_{y, -}|)

Equivalent Stress σ_v,Bach,- on Negative Surface Side

σ_{v, Bach, m} = \max (\frac{1 - ν}{2} |σ_{x, m} + σ_{y, m}| + \frac{1 + ν}{2} \sqrt{{(σ_{x, m} - σ_{y, m})}^{2} + 4 {τ_{xy, m}}^{2}}; ν |σ_{x, m} + σ_{y, m}|)

Membrane Equivalent Stress σ_v,Bach,m

Parent Chapter

Results by Surface