You need a section to describe the properties of a member: The section properties and the assigned material properties affect the stiffness of the member.
Not every section that has been defined needs to be used in the model. This way, you can model variants quickly, without deleting cross-sections. However, it is not possible to renumber the sections.
Name
You can define any name for the section and specify its cross-section properties. If the description matches an entry in the library, RFEM imports the stored properties. To select the cross-section from the library, click the
button at the end of the input line. The import of sections is described in the chapter Cross-Section Library.
For cross-sections from the library, the section properties are set by default and cannot be changed. The shear areas and the dimensions for non-uniform temperature loads are exceptions.
For a user-defined section name, you have to define all section properties manually. You can then use the cross-section to determine the internal forces and moments. However, the design of this cross-section is impossible, because no stress points can be defined.
Main
The Main tab manages the basic section parameters.
Material
It is necessary to assign a material to each cross-section. You can select it from the list of already defined materials. The buttons next to the text box allow you to select a material from the library or to define a new one (see the chapter Materials).
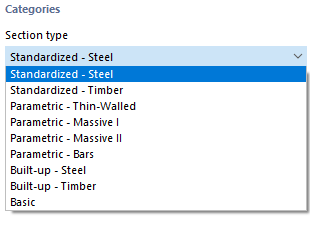
Categories
Section Type
A "Section type" is set by default, corresponding to the usual classifications of cross-sections from the library (see the chapter Cross-Section Library). User-defined cross-sections are assigned to the "Basic" type.
Manufacturing type
The production method of a cross-section is displayed for the cross-sections from the library. It controls certain design specifications; for example, the buckling curves of cold-formed hollow sections.
Options
Deactivate Shear Stiffness
Taking shear stiffness into account leads to an increase in deformation due to shear forces. Shear deformation plays a minor role in rolled and welded cross-sections. For solid and timber cross-sections, however, we recommend considering the shear stiffnesses for the deformation analysis.
Deactivate Warping Stiffness
The check box for considering warping stiffness is available if the Torsional Warping analysis add-on has been activated in the Base Data. In this case, you can control whether the warping stiffness of the cross-section is applied in the calculation with seven degrees of freedom.
Cross-Section Rotation
The section rotation describes the angle by which the cross-section is rotated. You can define rotation angle α' in the Section Rotation tab.
For unsymmetric cross-sections, this tab also provides options to "Mirror" the cross-section. This way, you can put an L-section in the correct position, for example.
When importing a cross-section from the library or RSECTION, you do not need to take care of the section rotation angle α'. RFEM imports the angle automatically. For user-defined cross-sections, however, you have to determine the principal axis angle manually and adjust the position using the section rotation.
Hybrid
The "Hybrid" option is available for cross-sections of the "Parametric – Massive II" type as well as for RSECTION cross-sections consisting of several materials. In the Hybrid tab, you can assign material properties to the components of built-up timber cross-sections.
Enter the "Reference Material"—one of the component materials—that you want to use to determine the ideal cross-section properties of the built-up section. The stiffness components of the structural elements are determined in relation to the reference material, taking into account the respective material properties. However, the choice of reference material has no effect on the stiffness of the overall section.
Thin-Walled Model
The "Thin-walled model" check box allows you to control the analysis according to which the cross-section properties are determined for cross-sections of the "Standardized – Steel" and "Parametric – Thin-Walled" type. For a massive section, for example, the shear areas and the torsional moment of inertia are determined according to a different method, since the analytical solution only applies to thin-walled sections.
US Notation for Section Properties
The symbols of the cross-section properties differ according to the European and American conventions. Mit dem Kontrollfeld können Sie steuern, ob beispielsweise die statischen Momente als S oder Q bezeichnet werden.
Stress Smoothing to Avoid Singularities
The stress smoothing is primarily suitable for composite timber sections in order to avoid singularities in the connection areas. In these areas, shear stresses often lead to stress peaks, which have an unfavorable effect on the design. This function allows you to achieve a better distribution of stresses.
Section properties
In diesem Abschnitt sind die wichtigsten Querschnittswerte angegeben. Weitere Kennwerte finden Sie im Register Querschnittswerte.
Sectional Areas
The cross-sectional areas are subdivided into the total area "Axial A", and the areas for "Shear Ay" and "Shear Az". The shear area Ay is related to the moment of inertia Iz; the shear area Az is related to Iy accordingly.
The following technical article provides information on determining shear areas:
https://www.dlubal.com/en/support-and-learning/support/knowledge-base/000966
The shear areas affect the shear deformation, which should be taken into account especially for short, massive members. If you change the shear areas, you should avoid extremely small values: The shear areas are included in the denominator of equations, so numerical problems may arise.
Area Moments of Inertia
The second moments of area define the section stiffness with regard to loading by moments: The torsional constant IT describes the stiffness against rotation about the longitudinal axis. The second moments of area Iy and Iz describe the stiffnesses against bending about the local axes y and z. Axis y is considered to be the "major" axis. The warping moment of inertia Iω is used to describe the resistance to warping.
For unsymmetric cross-sections, the second moments of area are displayed around the principal axes u and v of the cross-section. The local section axes are shown in the cross-section graphic.
You can adjust the sectional areas and moments of inertia using factors that you can define as section-specific "structural modifications" (see the chapter Structure Modifications).
Inclination of Principal Axes
The inclination of principal axes describes the position of the principal axes in relation to the standard principal axis system of symmetrical cross-sections. For unsymmetric cross-sections, this is angle α between axis y and axis u (clockwise positive). The principal axes are called y and z for symmetric cross-sections, and u and v for unsymmetric cross-sections (see the image Section Properties and Axes).
The principal axis inclination is determined according to the following equation:
| α | Principal axis angle |
| Iyz | Biaxial second moment of area |
| Iz | Moment of inertia about the z-axis |
| Iy | Moment of inertia about the y-axis |
The inclination of principal axes for sections from the library cannot be edited. However, you can rotate the section around a user-defined angle: Select the "Section rotation" check box in the "Options" section (see Section Rotation).
Dimensions (for Non-Uniform Temperature Loads)
The dimensions concerning width b and depth h of the cross-section are required for the calculation of temperature loads.
Section properties
In the Section Values tab, the properties of the cross-section are listed in detail.
The section properties of parametric cross-sections are determined using RSECTION.
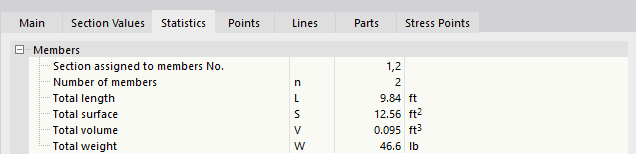
Statistics
The Statistics tab provides an overview of the members available in the model that use that cross-section. For example, you can use the "Total weight" indication for steel schedules or cost estimation.
Points
Die Geometrie des Querschnitts wird über Punkte definiert. Sie stellen auch die Grundlage für Linien dar.
Die Koordinaten der Definitionspunkte sind in einer Tabelle aufgelistet. Wenn Sie eine Zeile selektieren, wird dieser Punkt in der Querschnittsgrafik rot dargestellt. Bei dünnwandigen Querschnitten sind die Definitionspunkte auf den Mittellinien mit einem + Symbol gekennzeichnet. Generierte Kontrollpunkte für Bögen sind an einem Schloss-Symbol mit + zu erkennen. Die Punkte auf den Querschnittsrändern ergeben sich aus den Elementdicken.
Bei Bögen können Sie im Abschnitt 'Parameter' neben den Punktkoordinaten die Bogenparameter ablesen.
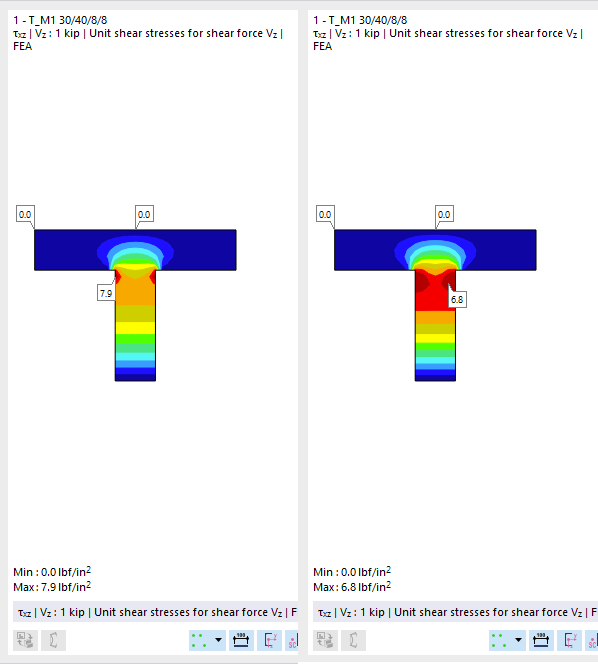
Stress points
Stress points are required to determine the stresses acting on the cross-section. All library sections are provided with stress points at the design-relevant locations of the cross-section.
Das Register Spannungspunkte besteht aus bis zu vier Unterregistern. Dort können Sie die Koordinaten der Spannungspunkte, die statischen Momente und Wölbordinaten mit den zugehörigen Dicken (bei dünnwandigen Querschnitten) sowie die Einheitsspannungen berechnet mit dünnwandiger Theorie TWA (bei dünnwandigen Querschnitten) und mit Finite-Elemente-Methode FEM ablesen.
You can check the cross-section distribution and stress curves in the cross-section graphic: Click in the column of the value, or select the type in the list below the graphic.