Considering creep

Considering creep

Creep describes the time-dependent deformation of the concrete with loading within a particular period of time. The essential influence values are similar to those of shrinkage. Additionally, the so-called "creep-producing stress" has a considerable effect on the creep deformations.

Attention must be paid to the load duration, the time of load application, as well as the extent of the loading. Creep is taken into account by the creep coefficient φ(t,t₀ ) at the point of time t.

You can activate creep in the material dialog box in the Time-Dependent Properties of Concrete section. Here, you can specify the age of concrete at the considered point of time and at the beginning of loading, the relative air humidity, and the type of cement. Based on these specifications, the program determines the creep coefficient φ.

Determination of creep coefficient

We now will briefly look at the determination of the creep coefficient φ according to EN 1992-1-1, clause 3.1.4. Using the following equations requires the creep-producing stress σ_c of the acting permanent load to not exceed the following value.

σ_c ≤ 0.45 · f_ckj
where
f_ckj - cylinder compressive strength of the concrete at the point of time when the creep-producing stress is applied

Under the assumption of a linear creep behavior (σ_c << 0.45 ⋅ f_ckj ), the concrete's creep can be determined by a reduction of the concrete's modulus of elasticity.

According to EN 1992-1-1, clause 3.1.4, the creep coefficient φ(t,t₀) at the analyzed point of time t can be calculated as follows.











The influence of the type of cement on the concrete's creep coefficient can be taken into account by modifying the load application age t₀ with the following equation:

Considering creep in the calculation

If the strains at the point of time t = 0 as well as at a later point of time t are known, it is possible to determine the creep coefficient φ for a calculational consideration in the model.

This equation is rearranged to the strain at the point of time t. Thus, we obtain the following relation, which is valid for uniform stresses:

For stresses higher than approximately 0.4 ⋅ f_ck, the strains increase disproportionately, resulting in the loss of the linearly assumed reference.

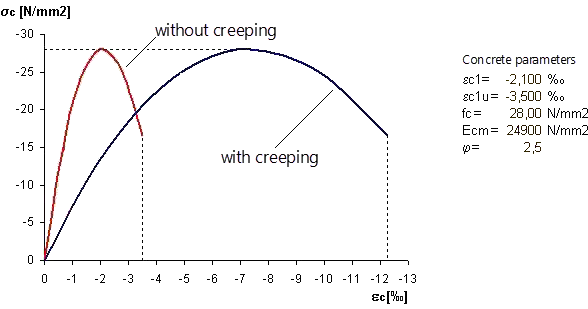
The calculation uses the solution allowed for practical construction purposes according to EN 1992-1-1, 5.8.6 (3). The stress-strain diagram of the concrete is distorted by the factor (1 + φ).

When taking creep into account, uniform creep-producing stresses are assumed during the period of load application, as can be seen in the figure above. Because of neglected stress redistributions, the deformation is slightly overestimated due to this assumption. The stress reduction without a change in strain (relaxation) is only taken into account to a limited degree in this model. If we assume a linear elastic behavior, a proportionality could be presumed and the horizontal distortion would also reflect the relaxation at a ratio of (1 + φ). This correlation, however, is lost for the nonlinear stress-strain relationship.

Thus, it becomes clear that this procedure must be understood as an approximation. Therefore, a reduction of the stresses due to relaxation as well as nonlinear creep cannot or can only be approximately represented.