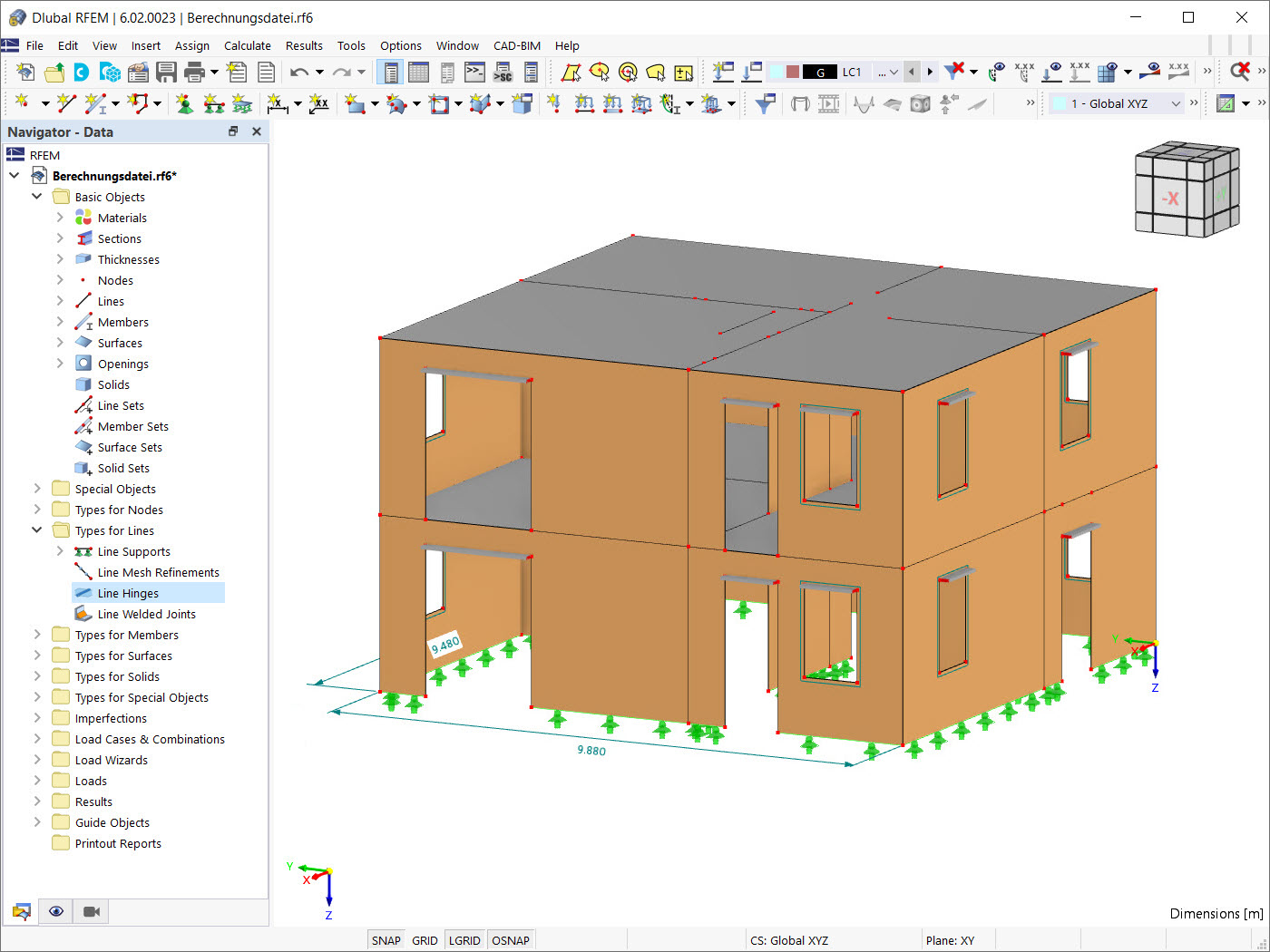
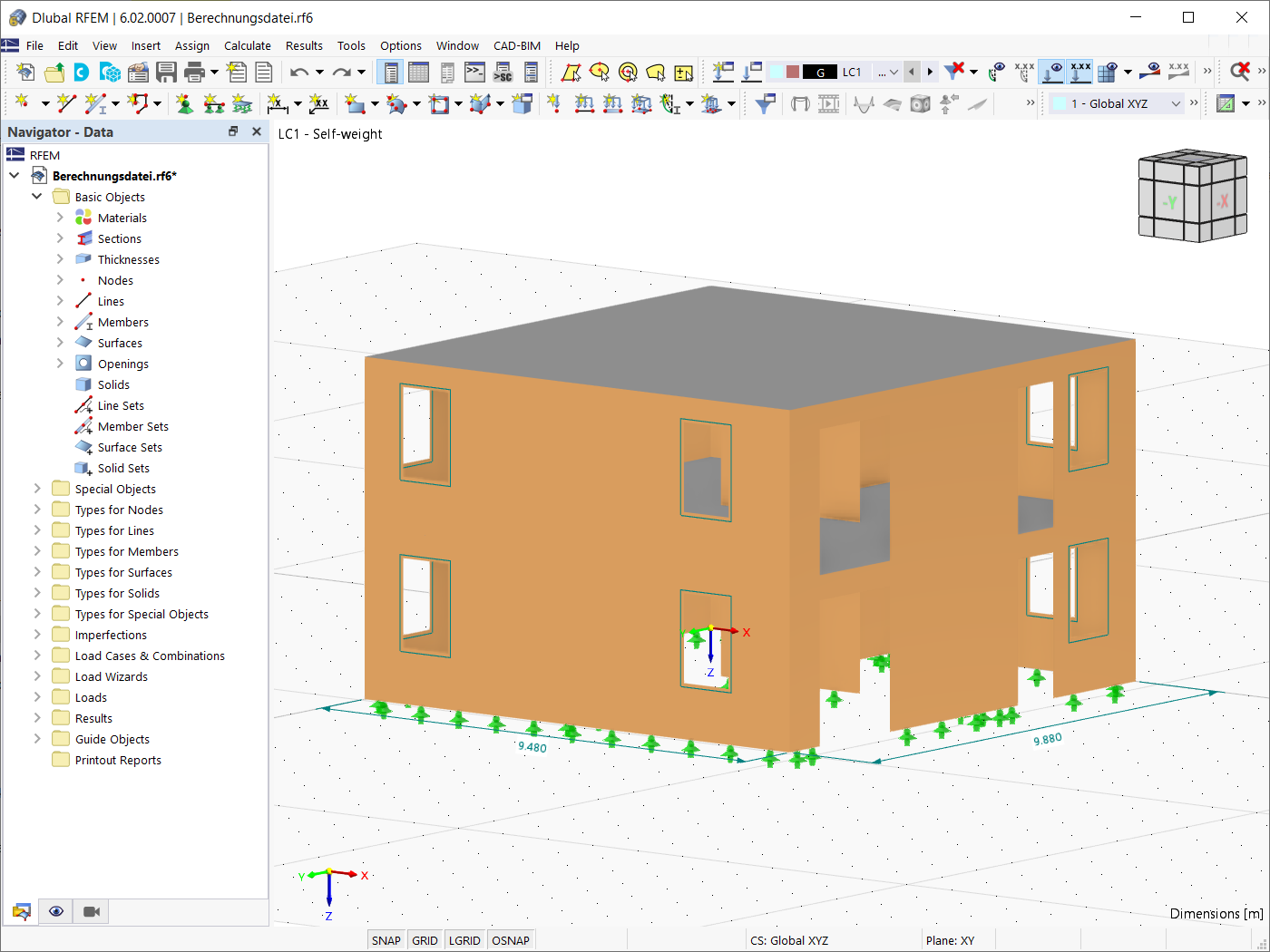
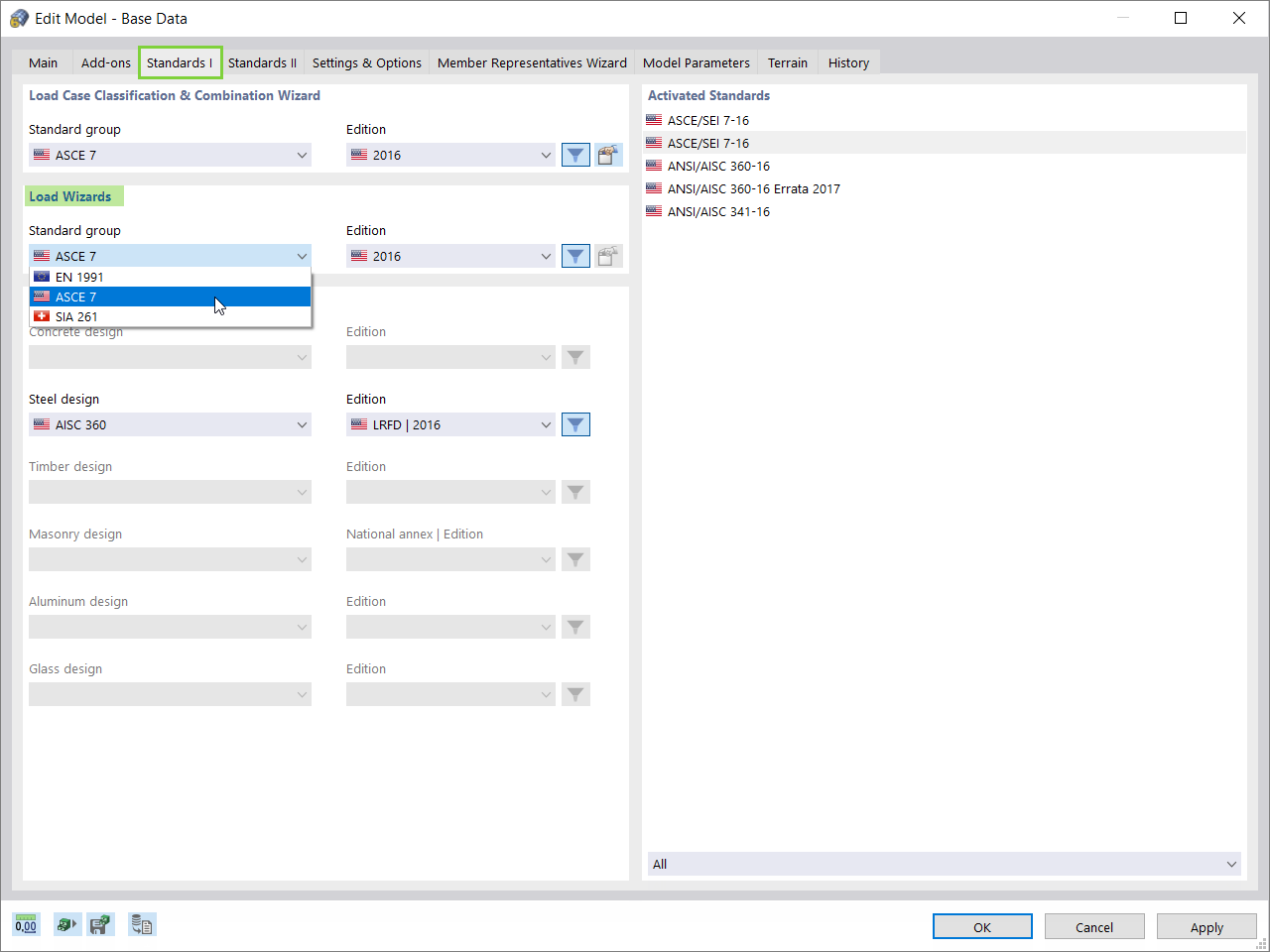
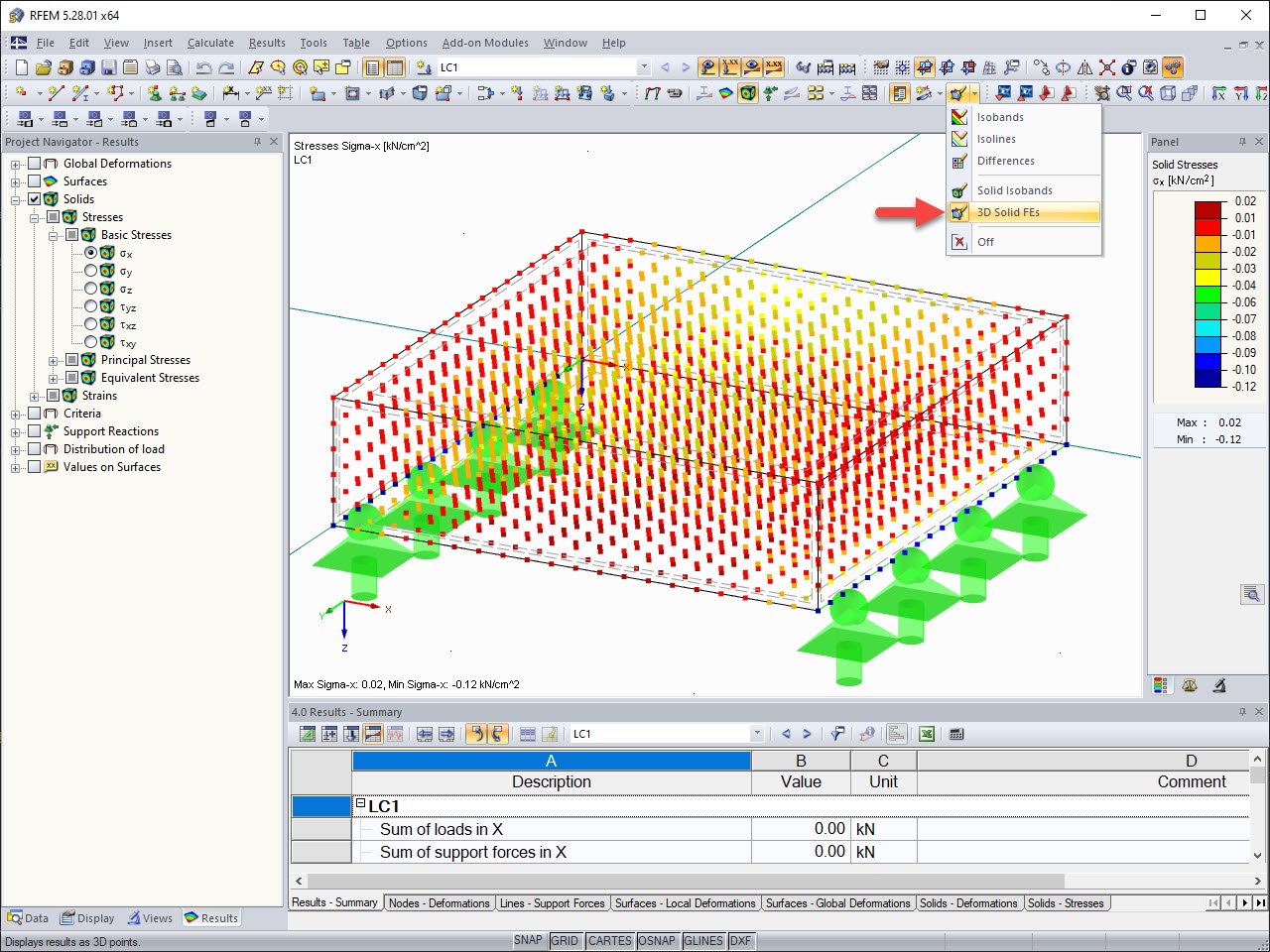
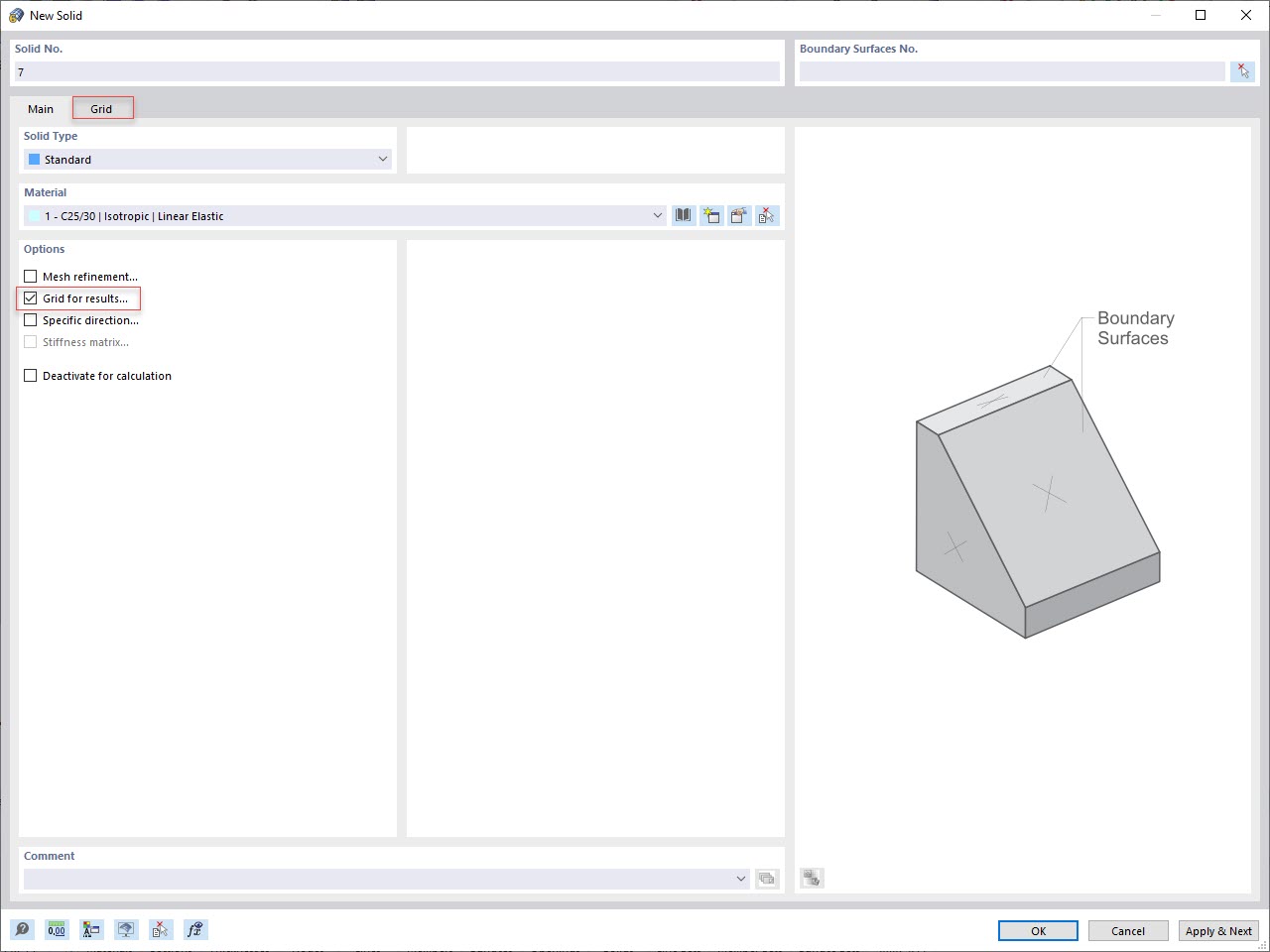
Flow and numerical models have specific characteristics that require expert evaluation. A common feature of nearly all computational methods is the iterative solution of nonlinear equations, with the number of time steps and iterations determined by convergence conditions.
One challenge in flow calculations is the potential propagation of errors, which can lead to the divergence of the computation. To prevent this, limiters for variables are often used, which must be set sufficiently far from the actual values. Physically impossible values, such as negative absolute temperatures, must be strictly excluded.
The most effective way to check for convergence is through graphical representation of the progress of steady-state target variables, such as means or standard deviations. Figure 6.7 exemplifies the progression of a target value over the number of iterations. It becomes clear that a coarse grid converges more quickly than a fine grid, although different final values may be reached. This visualization allows for quick and intuitive assessment of convergence and the grid-dependency of the results.

























.png?mw=512&hash=4a84cbc5b1eacf1afb4217e8e43c5cb50ed8d827)