888x

2024-01-15

VE0052 | Cantilever with Moment Loading at Free End

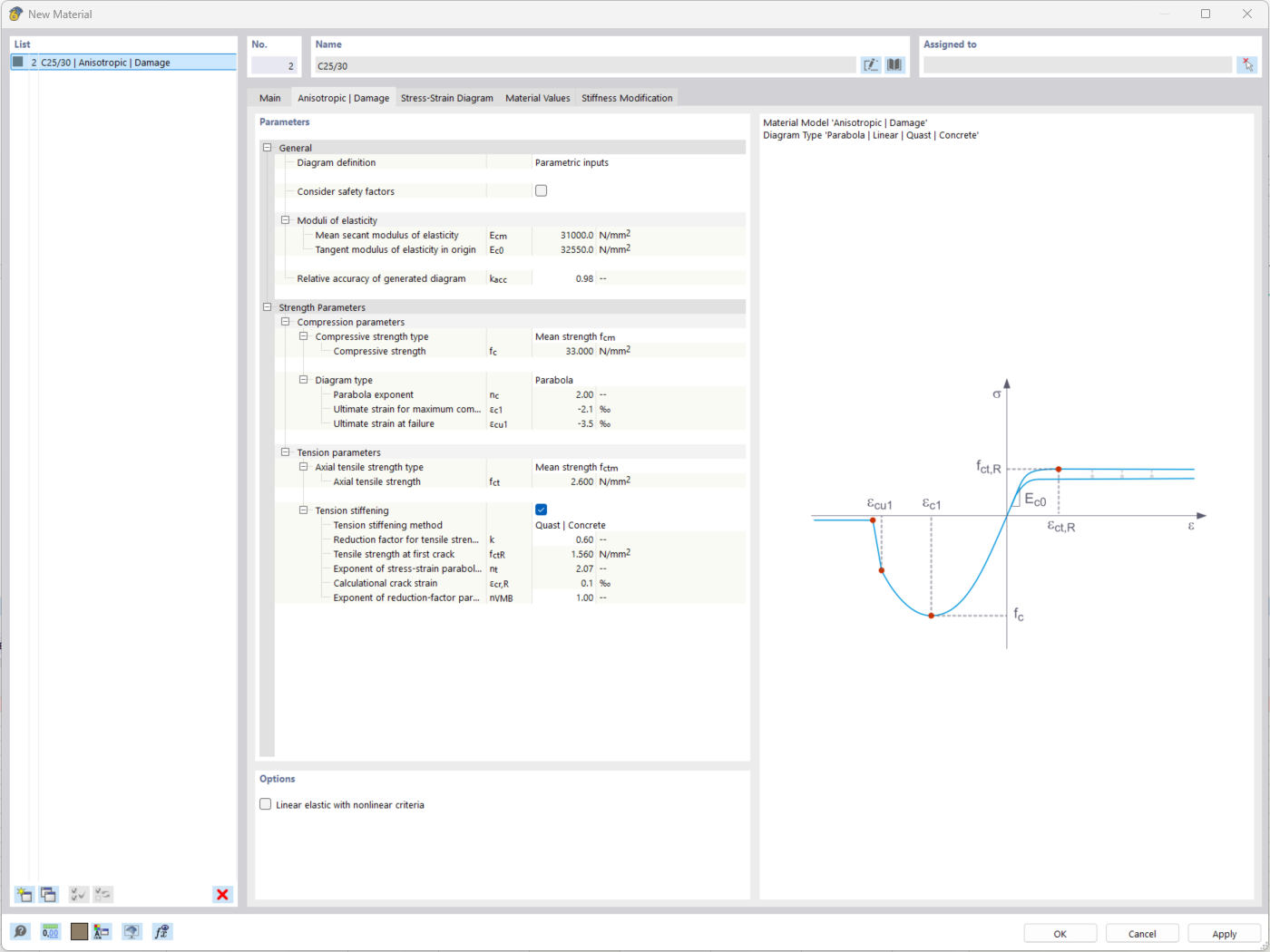
Description

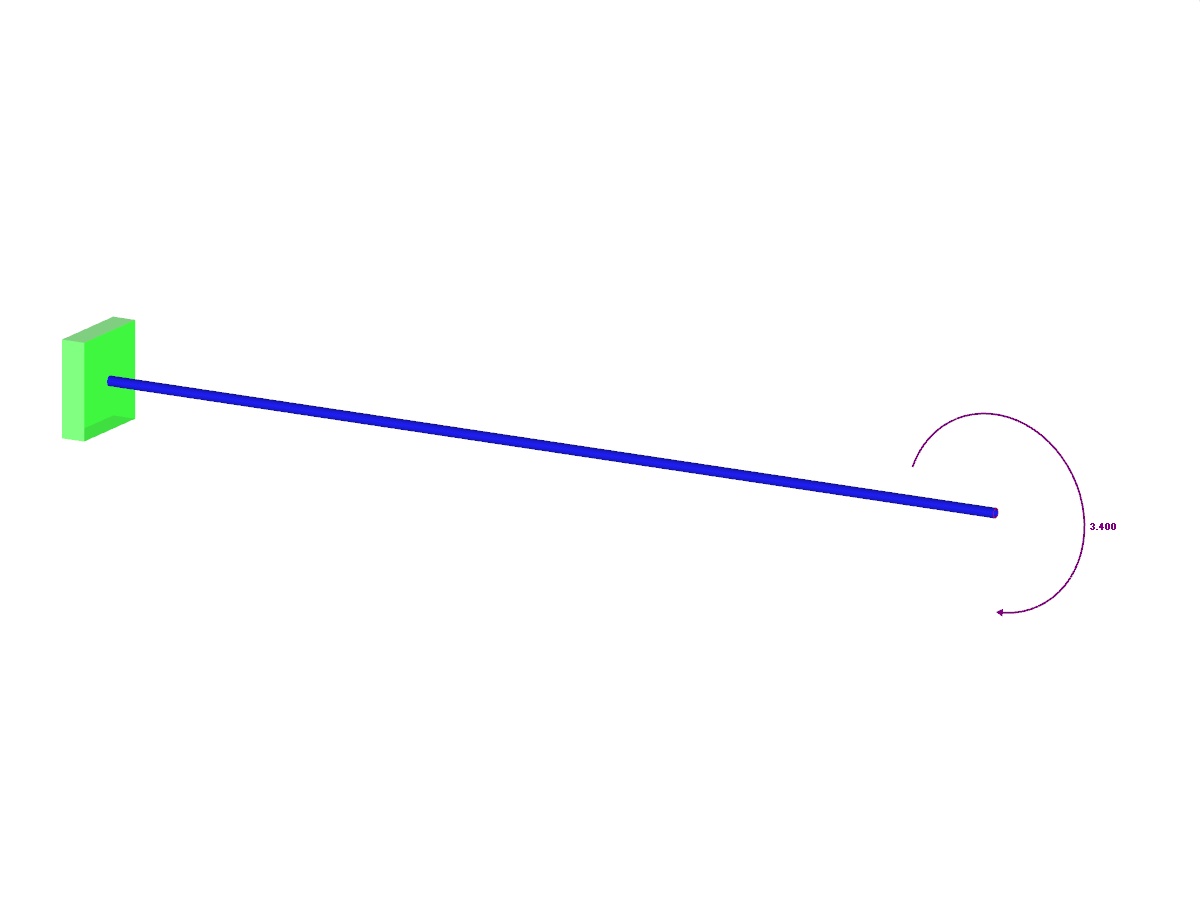
A cantilever is loaded by the moment M at its free end. Using the geometrically linear analysis and the large deformation analysis and neglecting beam's self-weight, determine the maximum deflections u_x and u_z at the free end. The verification example is based on the example introduced by Gensichen and Lumpe (see the reference).

Material	Steel	Modulus of Elasticity	E	210000.000	MPa
Material	Steel	Shear Modulus	G	81000.000	MPa
Geometry	Tube Cantilever	Length	L	4.000	m
		Diameter	d	42.400	mm
		Wall Thickness	t	4.000	mm
Load		Bending Moment	M	3.400	kNm

Cantilever with Moment Loading at Free End

Analytical Solution

Geometrically Linear Analysis

Considering the geometrically linear analysis, the problem can be solved according to the Euler-Bernoulli equation. For the given geometry, loading and boundary conditions, the result maximum deflection u_z,max is following:

$u_{z, \max} = \frac{{ML}^{2}}{2 {EI}_{y}}$

Maximum Deflection – Geometrically Linear Analysis

The deflection u_x,max considering geometrically linear analysis is zero.

Large Deformational Analysis

A beam in the large deformation analysis is described by the nonlinear differential equation and it is illustraded in the following figure.

$κ (x) = \frac{u_{z}'' (x)}{{[1 + (u_{z}' (x))^{2}]}^{\frac{3}{2}}} = - \frac{M}{{EI}_{y}}$

Differential Equation of Beam Deflection

Cantilever with Moment Loading at Free End – Large Deformation Analysis

The term on the right-hand side is constant and consequently the left-hand side, which is directly the beam curvature κ, is also constant. The only curve which has constant curvature is a circle, therefore, the solution to this problem is a circle arc of radius R.

$u_{x, \max} = Rsinα - L$

Maximum Deflection in x-Direction – Large Deformation Analysis

$u_{z, \max} = R (1 - cosα)$

Maximum Deflection in z-Direction – Large Deformation Analysis

R is the radius of the circular arc. The angle of the circular arc α equals to α=L/R.

RFEM and RSTAB Settings

Modeled in RFEM 5.05, RSTAB 8.05 and RFEM 6.01, RSTAB 9.01
The element size is l_FE= 0.400 m
The number of increments is 5
Isotropic linear elastic material model is used
Shear stiffness of members is activated
Member division for large deformation or post-critical analysis is activated

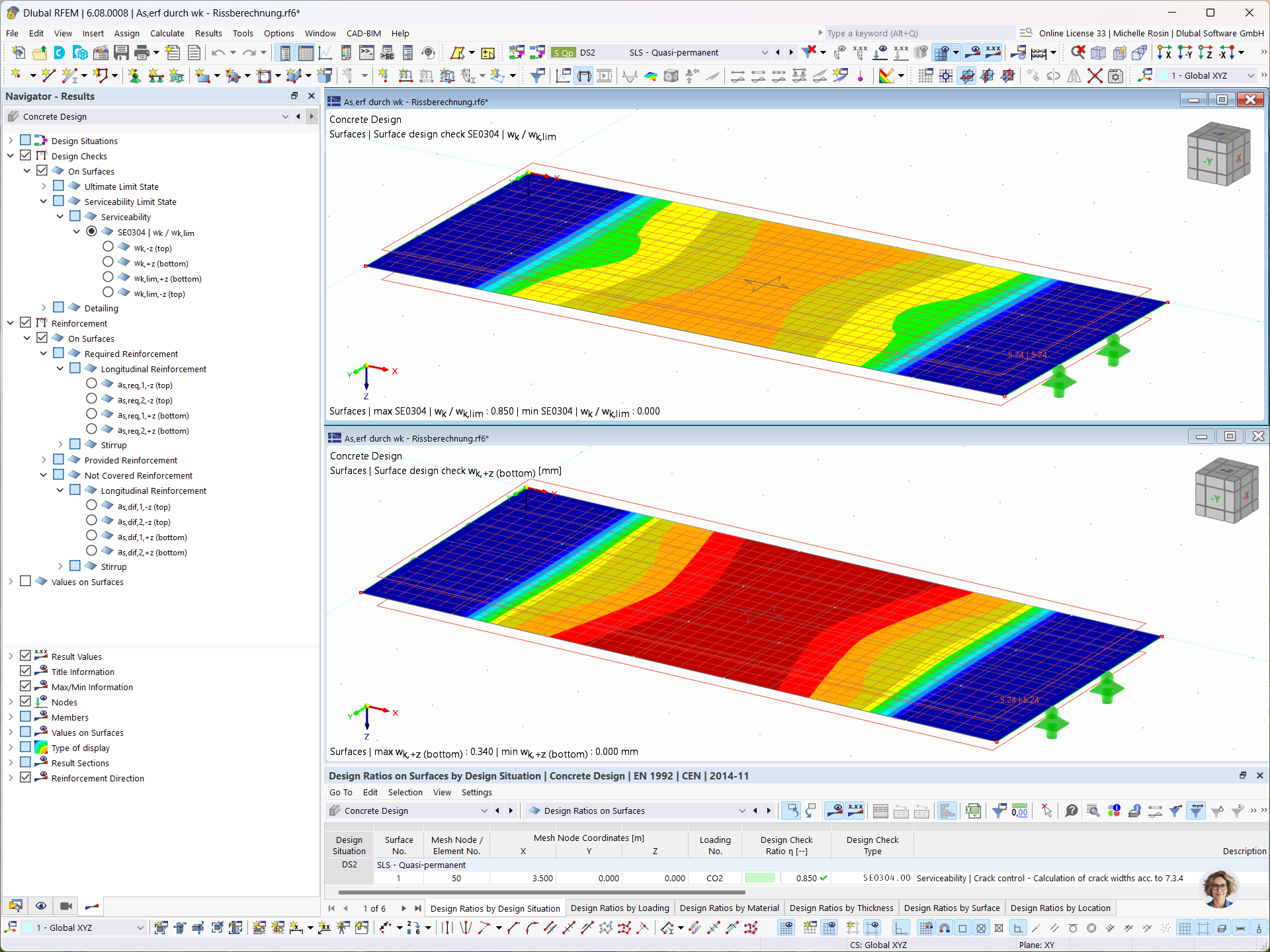
Results

u_{x, max} [m]	Analytical Solution	RFEM 6	Ratio	RSTAB 9	Ratio
Geometrically Linear Analysis	0.000	0.000	-	0.000	-
Large Deformation Analysis	-0.337	-0.336	0.997	-0.336	0.997

u_{z, max} [m]	Analytical Solution	RFEM 6	Ratio	RSTAB 9	Ratio
Geometrically Linear Analysis	1.441	1.441	1.000	1.441	1.000
Large Deformation Analysis	1.379	1.380	1.001	1.380	1.001

u_{x, max} [m]	Analytical Solution	RFEM 5	Ratio	RSTAB 8	Ratio
Geometrically Linear Analysis	0.000	0.000	-	0.000	-
Large Deformation Analysis	-0.337	-0.338	1.003	-0.337	1.000

u_{z, max} [m]	Analytical Solution	RFEM 5	Ratio	RSTAB 8	Ratio
Geometrically Linear Analysis	1.441	1.441	1.000	1.441	1.000
Large Deformation Analysis	1.379	1.380	1.001	1.380	1.001

396x

Verification Example 000052 | 1

References

LUMPE, G. and GENSITEN, V. Evaluation of Linear and Nonlinear Member Analysis in Theory and Software: Test examples, causes of failure, detailed theory. Ernest.

Gensichen

;