Turbulence modeling plays a vital role in computational fluid dynamics (CFD) by aiming to predict the behavior of turbulent flows. These models are crucial for creating efficient and safe engineering applications, such as analyzing and designing wind-structure interactions. There are several approaches to turbulence modeling, with three widely used models being the Reynolds-Averaged Navier-Stokes (RANS), Unsteady Reynolds-Averaged Navier-Stokes (URANS), and Delayed Detached Eddy Simulation (DDES). Each of these models has distinct characteristics and specific applications.
RWIND employs URANS and DDES turbulence models as applicable transient turbulence models in the structural engineering field. These models are chosen for their ability to balance computational efficiency and accuracy, making them practical for a wide range of structural engineering applications. URANS offers a moderate computational cost while capturing large-scale transient phenomena, whereas DDES combines the strengths of both RANS and Large Eddy Simulation (LES) to provide detailed resolution of complex turbulent structures. By integrating these models, RWIND enhances its capability to simulate the behavior of wind-structure interactions, ensuring both efficiency and precision in its analyses.
URANS (Unsteady Reynolds-Averaged Navier-Stokes)
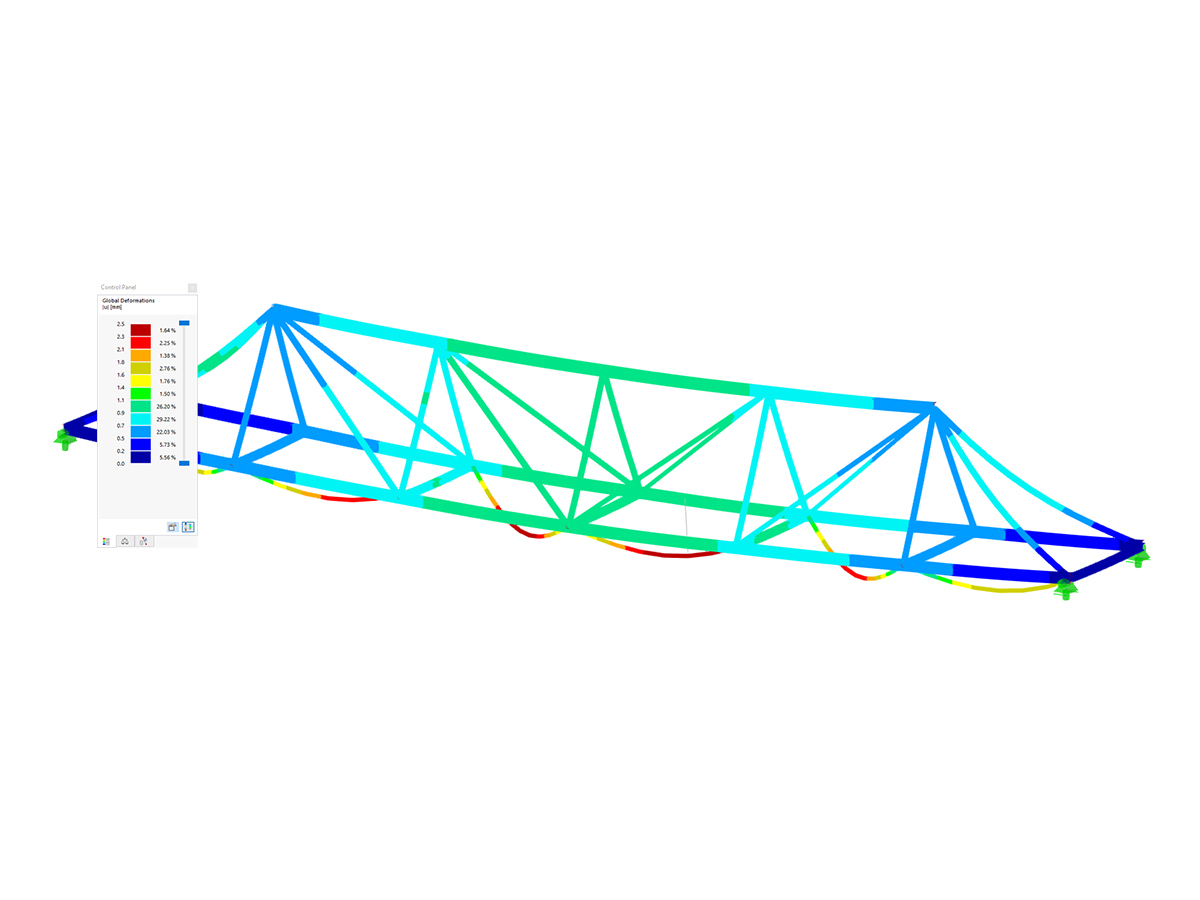
URANS builds on the RANS method by accommodating time-dependent changes in the flow field, allowing it to capture unsteady effects of wind load. This allows URANS to model larger-scale transient flow features and oscillatory behaviors, such as vortex shedding from building corners. Although URANS offers improvements over RANS in capturing unsteadiness, it still uses eddy-viscosity models that may not adequately resolve finer turbulent structures (Image 1).
DDES (Delayed Detached Eddy Simulation)
DDES is a hybrid method that integrates RANS and Large Eddy Simulation (LES) techniques. In regions with an attached boundary layer, DDES functions like a RANS model, ensuring computational efficiency. In areas where the flow detaches and larger turbulent structures prevail, DDES switches to an LES mode for more accurate resolution. This approach is particularly effective for complex flows involving separation, reattachment, and wake regions, such as around building edges and corners. DDES strikes a good balance between computational cost and accuracy, making it suitable for simulating high Reynolds number flows with significant unsteady and separated regions (Image 2).
Advantages and Disadvantages of URANS and DDES
URANS Advantages
- Time-Dependent Capabilities: URANS can capture unsteady phenomena and transient flow features, such as vortex shedding, which RANS cannot.
- Moderate Computational Cost: While more computationally intensive than RANS, URANS is still relatively efficient compared to more complex models like LES or DDES.
- Practical for Engineering Applications: URANS is suitable for many practical engineering systems where capturing large-scale unsteady behaviors is important.
URANS Disadvantages
- Limited Accuracy for Complex Flows: URANS may not accurately predict finer turbulent structures due to its reliance on eddy-viscosity models.
- Still Averaged in Time: Although less strictly than RANS, URANS still averages the flow over time, which can smooth out some important transient details.
- Less Effective for Strongly Separated Flows: URANS might struggle with flows that have significant separation and reattachment, as it is not as refined as LES or hybrid methods in these scenarios.
DDES Advantages
- Hybrid Approach: DDES combines the strengths of RANS and LES, allowing for efficient simulation of both attached boundary layers and detached turbulent regions.
- Accuracy in Complex Flows: DDES can more accurately resolve large turbulent structures and complex flow behaviors such as separation, reattachment, and wakes, providing better predictions for high Reynolds number flows.
- Computational Efficiency: By switching between RANS and LES modes, DDES maintains a balance between computational cost and simulation accuracy, making it more efficient than full LES.
DDES Disadvantages
- Higher Computational Cost than URANS: DDES is more computationally intensive than URANS due to the need for LES calculations in certain flow regions.
- Complex Implementation: The hybrid nature of DDES requires careful implementation and calibration to ensure the model transitions smoothly between RANS and LES regions.
- Sensitivity to Grid Resolution: DDES performance is highly dependent on the quality and resolution of the computational grid, particularly in regions where the model switches from RANS to LES. Despite its advantages in computational efficiency and accuracy, particularly for high-Reynolds-number flows, DDES faces a significant challenge in the form of the "Grey Area." This transitional region, where the flow shifts from RANS to LES, often experiences a turbulence deficit due to underdeveloped turbulence structures. This deficit can lead to inaccuracies that compromise the entire simulation. To mitigate this issue, researchers use strategies such as refining the mesh, adjusting blending functions, and improving turbulence generation at the interface. Addressing the Grey Area is crucial for ensuring the accuracy and reliability of DDES in complex flow simulations (Image 3).
Generally, steady-state simulations provide insight into the average wind loads by assuming a constant flow condition as one time step, which is valuable for some engineering applications where wind conditions are relatively stable. On the other hand, transient simulations offer a time-dependent analysis that captures these fluctuating wind conditions, making it possible to predict the effects of wind load more realistic during a defined time domain, and it also leads to capturing critical wind pressure values (which may miss in steady-state simulation) in order to structural design, specifically in highly turbulent regions or suction areas (Image 4).
Conclusion
In summary, URANS is advantageous for capturing large-scale unsteady phenomena at a moderate computational cost but may fall short in accuracy for complex turbulent flows. DDES offers a more accurate representation of such flows by combining RANS and LES methods, though it comes with higher computational demands and complexity.




















































_1.jpg?mw=350&hash=ab2086621f4e50c8c8fb8f3c211a22bc246e0552)


.png?mw=600&hash=49b6a289915d28aa461360f7308b092631b1446e)