17201x

001497

Dipl.-Ing. (FH) Walter Fröhlich

2017-12-12

Calculation Example of Rigid End Plate Connection According to EN 1993-1-8

In this example, the design resistance of an end plate according to EN 1993-1-8 [1] is to be determined; the other components are not described here. To check the results, the dimensions of the connection IH 3.1 B 30 24 of Typified Connections [2] were used. S 235 material and bolts with strength 10.9 are used.

Calculation Example

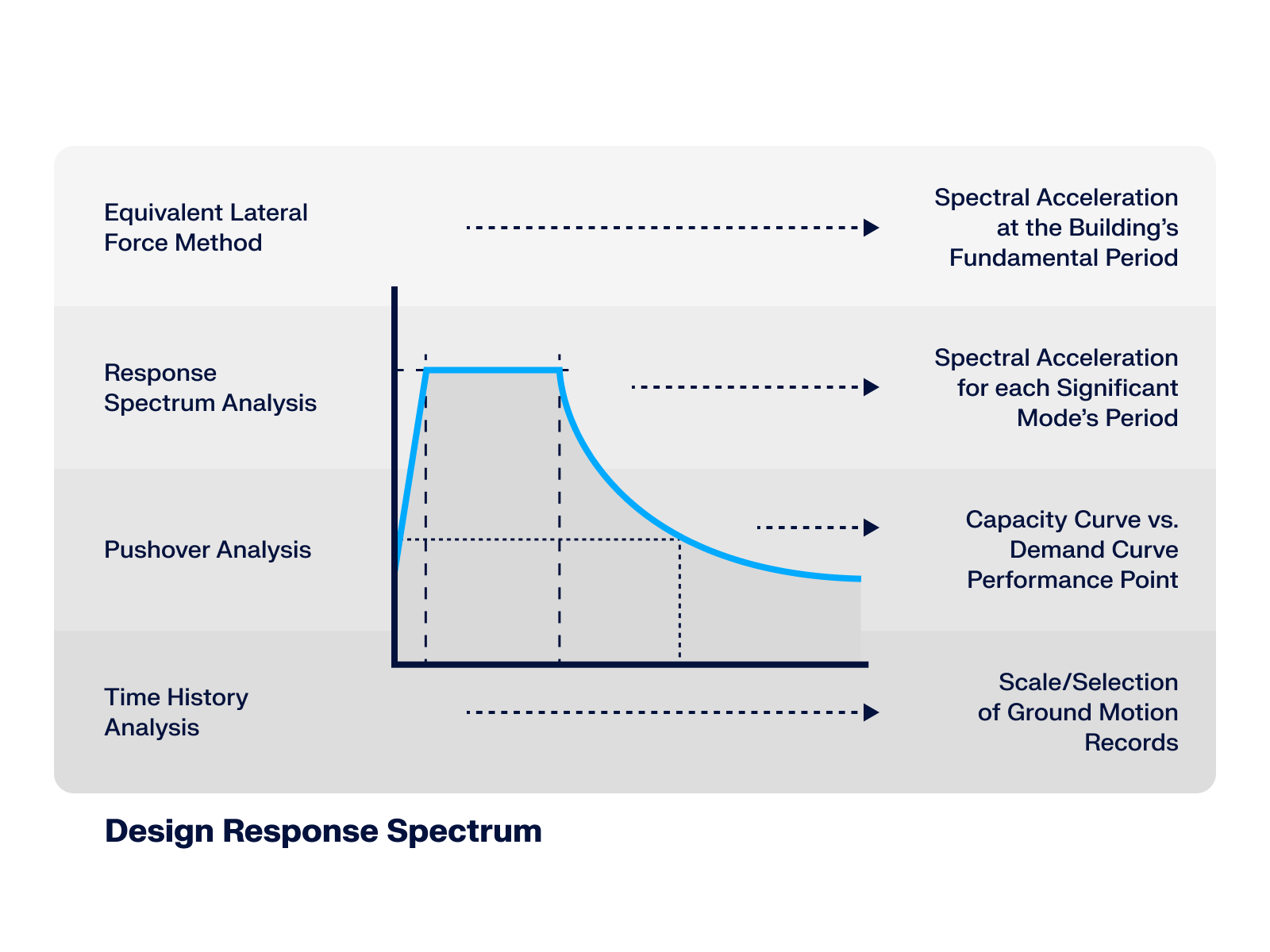
Determining Effective Lengths

First, it is necessary to determine the effective lengths of the stub flanges according to Table 6.6. The bottom bolt row has barely an effect on the compression flange due to the very small lever arm, and is therefore neglected. Since both upper bolt rows are divided by the tension flange of the beam, the bolt rows must only be considered separately. Thus, you can avoid group failure of the bolt rows. The calculation of the effective lengths requires the parameters e, m, ex, m_x, m₂, b_p, w.

Parameters for Effective Lengths (Source: [1])

For this example, the following values have been calculated:

\begin{array}{l} e = 75 mm \\ m = \frac{300 - 13.5}{2} - 75 - 0.8 \cdot 5 \cdot \sqrt{2} = 62.6 mm \\ e_{x} = 35 mm \\ m_{x} = 50 - 0.8 \cdot 9 \cdot \sqrt{2} = 39.8 mm \\ m_{2} = 125 - 50 - 24 - 0.8 \cdot 9 \cdot \sqrt{2} = 40.8 mm \\ b_{p} = 300 mm \\ w = 150 mm \end{array}

Formula 1

In the case of the effective lengths, a distinction is made between circular and non-circular yield line patterns. The linear yield line pattern requires value α from Figure 6.11. The input values for this are based on the relation of the lever arms to the girder web (λ₁) or to the girder flange (λ₂) to the total width of the T-stub flange. The values for α between two diagrams in Figure 6.11 may be linearly interpolated.

\begin{array}{l} λ_{1} = \frac{62.6}{62.6 75} = 0.45 \\ λ_{2} = \frac{40.8}{62.6 75} = 0.30 \\ α \approx 6.65 \end{array}

Formula 2

Determination of α-value (Source: [1])

Using these input values, the effective lengths are determined according to Table 6.6 as follows.

Circular yield line pattern for the outer bolt row:

l_{eff, cp, a} = \min \{\begin{matrix} 2 \cdot π \cdot 39.8 \\ π \cdot 39.8 150 \\ π \cdot 39.8 2 \cdot 75 \end{matrix}\} = 250.1 mm

Formula 3

Circular yield line pattern for the inner bolt row:
l_eff,cp,i = 2 ∙ π ∙ 62.6 = 393.3 mm

Non-circular yield line pattern for the outer bolt row:

l_{eff, nc, a} = \min \{\begin{matrix} 4 \cdot 39.8 1.25 \cdot 35 \\ 75 2 \cdot 39.8 0.625 \cdot 35 \\ 0.5 \cdot 300 \\ 0.5 \cdot 150 2 \cdot 39.8 0.625 \cdot 35 \end{matrix}\} = 150.0 mm

Formula 4

Non-circular yield line pattern for the inner bolt row:
l_eff,nc,i = 6.65 ∙ 62.6 = 416.3 mm

To determine the design resistance in Failure Mode 1, that is with pure flange yielding, the shorter length of both yield line patterns is used. When determining the design resistance in Failure Mode 2, that is bolt failure with simultaneous flange yielding, the non-circular yield line pattern can only occur.

This results in the following effective lengths.

Outer bolt row:
l_eff,1,a = 150 mm
l_eff,2,a = 150 mm

Inner bolt row:
l_eff1,i = 393.3 mm
l_eff2,i = 416.3 mm

Check if Prying Forces May Develop

Before the design resistance of the end plate in Failure Mode 1 is determined, it must be checked whether prying forces may develop. Since this allows you to achieve higher design resistance values, the dimensions and thickness of the grip package should always be chosen or changed in a way so that the equation L_b < L_b* is fulfilled. L_b is the bolt elongation length, taken equal to the grip length (total thickness of the material and washers), plus half the sum of the height of the bolt head and the height of the nut.

L_{b} * = \frac{8.8 \cdot m^{3} \cdot A_{s}}{l_{eff 1} \cdot t_{f} ³}

Formula 5

The grip length, assuming that a symmetrical beam joint is applied, results in:
L_b = 2 ∙ 25 + 2 ∙ 4 + 0.5 ∙ 19 + 0.5 ∙ 15 = 75 mm

L_b* must be determined separately for the outer and the inner bolt rows.

Outer bolt row:

L_{b} * = \frac{8.8 \cdot 39 . 8^{3} \cdot 353}{150 \cdot 25 ³} = 83.6 mm

Formula 6

Inner bolt row:

L_{b} * = \frac{8.8 \cdot 62 . 6^{3} \cdot 353}{393.3 \cdot 25 ³} = 124 mm

Formula 7

Therefore, prying forces may develop in both bolt rows.

Design Resistance of T-Stub Flanges

For the "complete yielding of the flange" failure mode, Method 1 of EN 1993-1-8 is used in this example. The tension resistance of both T-stub flanges is determined as follows.

\begin{array}{l} F_{T, 1, Rd} = \frac{4 \cdot M_{pl, 1, Rd}}{m} \\ where \\ M_{pl, 1, Rd} = \frac{0.25 \cdot l_{eff, 1} \cdot t_{f} ² \cdot f_{y}}{γ_{M 0}} \\ m = 39.8 mm for the outer bolt row \\ m = 62.6 mm for the inner bolt row \\ M_{pl, 1, Rd} = \frac{0.25 \cdot 15.0 \cdot 2.52 \cdot 23.5}{1.0} = 550.78 kNcm for the outer bolt row \\ M_{pl, 1, Rd} = \frac{0.25 \cdot 39.33 \cdot 2.52 \cdot 23.5}{1.0} = 1, 444.15 kNcm for the inner bolt row \\ F_{T, 1, Rd} = \frac{4 \cdot 550.78}{3.98} = 553.55 kN for the outer bolt row \\ F_{T, 1, Rd} = \frac{4 \cdot 1, 444.15}{6.26} = 922.78 kN for the inner bolt row \end{array}

Formula 8

"Bolt failure with yielding of the flange" failure mode:

\begin{array}{l} F_{T, 2, Rd} = \frac{2 \cdot M_{pl, 2, Rd} n \cdot {ΣF}_{t, Rd}}{m n} \\ where \\ M_{pl, 2, Rd} = \frac{0.25 \cdot l_{eff, 2} \cdot t_{f} ² \cdot f_{y}}{γ_{M 0}} \\ {ΣF}_{t, Rd} = ΣTension resistance of bolts \\ n = e_{\min} < 1.25 \cdot m \\ e_{\min} = 35 mm for the outer bolt row \\ e_{\min} = 75 mm for the inner bolt row \\ m = 39.8 mm for the outer bolt row \\ m = 62.6 mm for the inner bolt row \\ {ΣF}_{t, Rd} = \frac{2 \cdot k_{2} \cdot f_{ub} \cdot A_{S}}{γ_{M 2}} = \frac{2 \cdot 0.9 \cdot 100 \cdot 3.53}{1.25} = 508.32 kN \\ The punching force has been checked but is not governing . \\ M_{pl, 2, Rd} = \frac{0.25 \cdot 15.0 \cdot 2.52 \cdot 23.5}{1.0} = 550.78 kNcm for the outer bolt row \\ M_{pl, 2, Rd} = \frac{0.25 \cdot 41.63 \cdot 2.52 \cdot 23.5}{1.0} = 1, 528.60 kNcm for the inner bolt row \\ F_{T, 2, Rd} = \frac{2 \cdot 550.78 3.5 \cdot 508.32}{3.98 3.5} = 385.12 kN for the outer bolt row \\ F_{T, 2, Rd} = \frac{2 \cdot 1, 528.60 7.5 \cdot 508.32}{6.26 7.5} = 499.24 kN for the inner bolt row \end{array}

Formula 9

Governing the Design Resistance of T-Stub Flanges

Failure Mode 2 governs for both bolt rows.

Outer bolt row: 385.12 kN

Inner bolt row: 499.24 kN

Moment Resistance of the Joint

The calculated design resistance values of the individual bolt rows now only have to be multiplied by the respective lever arm to the compression point.

The lever arms are
438 mm for the outer bolt row,
313 mm for the inner bolt row.

Thus, the design moment resistance of the joint results in
M_Rd = 385.12 ∙ 0.438 + 499.24 ∙ 0.313 = 324.95 kNm.

Load-Bearing Capacities of Bolt Rows and Corresponding Lever Arms

Comparison of Results

If this joint is calculated as a rigid frame joint in RF-/FRAME-JOINT Pro, the resulting design resistance of the end plate is 319.79 kNm. According to Typified Connections [2], the design resistance is 331.3 kNm, which corresponds relatively accurately to the manual calculation.

Ultimate Limit State in RF-/FRAME-JOINT Pro

Author

Mr. Fröhlich provides technical support to our customers and is responsible for development in the area of reinforced concrete structures.

Links

Steel Connections

References

Eurocode 3: Bemessung und Konstruktion von Stahlbauten - Teil 1-8: Bemessung von Anschlüssen; EN 1993-1-8:2005 + AC:2009
Weynand, K., & Oerder, R. (2013). Typisierte Anschlüsse im Stahlhochbau nach DIN EN 1993-1-8. Duesseldorf: Stahlbau.

Downloads

RFEM Model File

1.16 MB

;