Question:
Why do I obtain great differences in results for both the deformation and the bending moments when applying inclination using imperfection to a single member and an articulated set of members with the same total length?
Answer:
Imperfections are considered for the calculation with equivalent member loads. The applied force transverse to the longitudinal direction of the member results from the multiplication of an axial force and an angle.
In the case of a variable axial force distribution, the average per member is used.
Particularly in the case of loading due to an increasing normal force (for example, from dead load), large differences may occur, since the applied force has a significantly longer lever arm in the case of a single member.
If a vertical nodal load in combination with the imperfection is only applied to the column head, the results of the member and the set of members are identical.
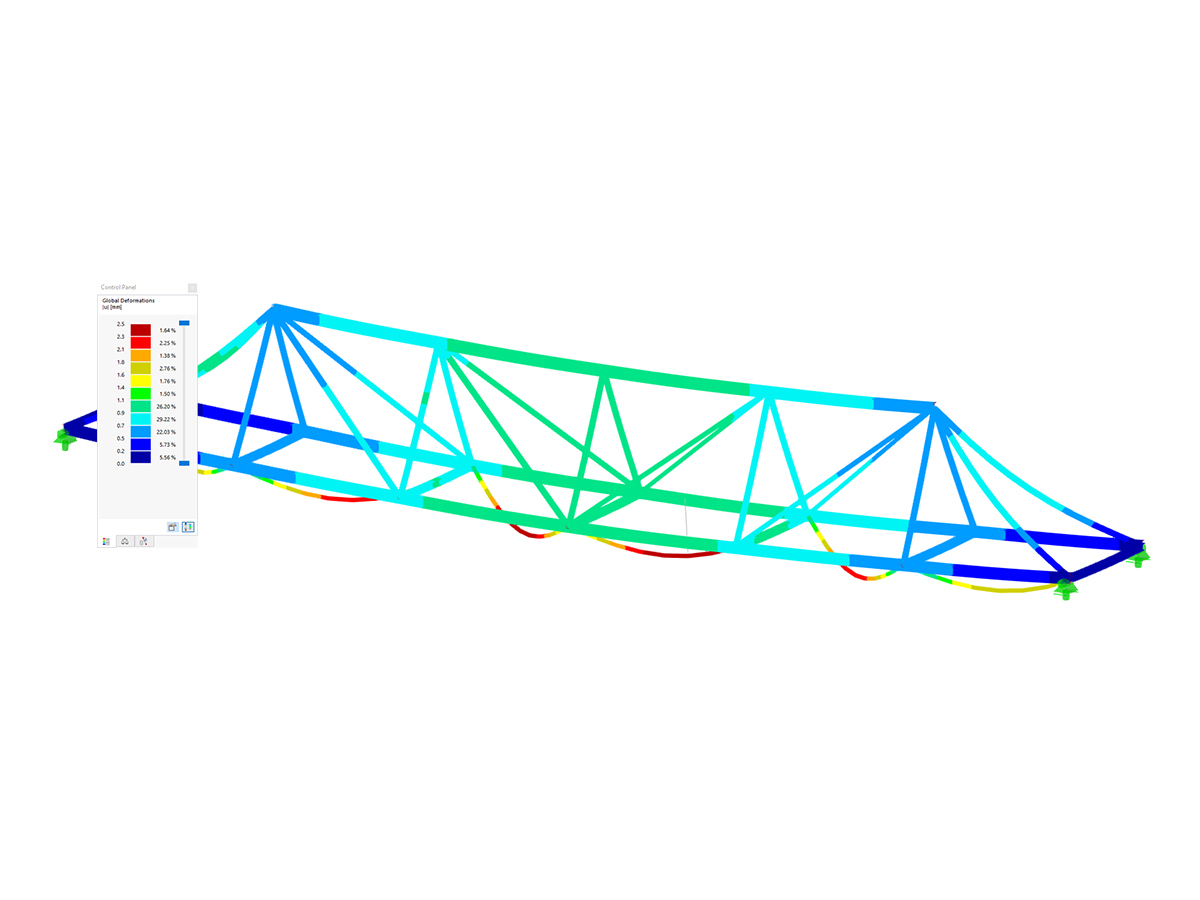
In the following, there is a simple example with a member and a set of four individual members with a total length of 8.00 m.
In order to avoid the calculation abort due to instability (by the iterative determination of the normal force to be applied), the absolute inclination in LC2 with 200 mm and a linearly variable load (to increase the effect) in LC1 from 2.5 kN/m to 0.00 kN/m were applied.
The imperfection load to be applied is obtained using the "Result Diagrams on Member" dialog box and the area-wise linear smoothing option for every 2.00 m and applied as a vertical load with changing sign. The horizontal loads result from the multiplication of the vertical load by (200 mm / 8,000 mm)
These equivalent loads were then applied to the members as nodal loads in LC4 and compared to the results of CO1.
The very small differences in the results are caused by rounding errors and the calculation according to the second-order analysis. Analysis



















.png?mw=350&hash=c6c25b135ffd26af9cd48d77813d2ba5853f936c)





















.png?mw=350&hash=dc38fc2ddc3754a07164885d9707318b00be194d)








_1.jpg?mw=350&hash=ab2086621f4e50c8c8fb8f3c211a22bc246e0552)





-querkraft-hertha-hurnaus.jpg?mw=350&hash=3306957537863c7a7dc17160e2ced5806b35a7fb)


.png?mw=600&hash=49b6a289915d28aa461360f7308b092631b1446e)