Se va a diseñar un pilar estructural de 10 pies, nominal 8 pulgadas ⋅ 8 pulgadas de cedro de Alaska con una carga axial de 30,00 kips. The goal of this analysis is to determine the adjusted compression factors and adjusted compressive design value of the column. Se asume una duración normal de la carga y apoyos articulados fijos en ambos extremos de la barra. The loading criteria are simplified for this example. Se puede hacer referencia a los criterios de carga normales en el apartado 1.4.4 [1]. In Image 01 and 02 is a diagram of the simple column and section properties respectfully.
Propiedades del pilar
The cross-section used in this example is an 8 inch square post. The cross-section properties of the timber column are shown below:
b = 7.50 in, d = 7.50 in, L = 10.00 ft
Área de la sección bruta:
Ag = b ⋅ d = 7.50 in ⋅ 7.50 in = 56.25 in2
Módulo resistente:
Momento de inercia:
The material used is "Alaska Cedar, 5"x5" and Larger, Beam and Stringer, Select Structural". Las propiedades del material son las siguientes:
Reference compression design value:
Fc = 925 psi
Módulo de elasticidad mínimo:
Emin = 440 ksi
Column Adjustment Factors
For the design per the 2018 NDS standard and the ASD method, stability factors (or adjustment factors) must be applied to the compressive design value (fc). This will ultimately provide the adjusted compressive design value (F'c). The factor F'c is calculated with the following equation, highly dependent on the listed adjustment factors from Table 4.3.1 [1]:
F'c = Fc ⋅ CD ⋅ CM ⋅ Ct ⋅ Cf ⋅ Ci ⋅ CP
A continuación, se determina cada coeficiente de ajuste:
CD – The load duration factor is implemented to take into account different periods of loading. La nieve, el viento y los terremotos se tienen en cuenta con CD. Este factor se debe multiplicar por todos los valores de cálculo de referencia excepto el módulo de elasticidad (E), el módulo de referencia de elasticidad para cálculos de estabilidad de barras y pilares (Emín) y los esfuerzos de compresión perpendiculares a la fibra (Fc) basados en el apartado 4.3.2 [1]. CD en este caso se establece en 1.00 según el apartado 2.3.2 [1] assuming a load duration of 10 years.
CM – The wet service factor references design values for structural sawn lumber based on moisture service conditions specified in Sec. 4.1.4 [1]. En este caso, según el apartado 4.3.3 [1], CM is set to 0.910.
Ct – The temperature factor is controlled by a member's sustained exposure to elevated temperatures up to 150 degrees Fahrenheit. Todos los valores de cálculo de referencia se multiplicarán por Ct. Utilizing Table 2.3.3 [1], Ct is set to 1.00 for all reference design values, assuming temperatures are equal to or lesser than 100 degrees Fahrenheit.
CF – The size factor for sawn lumber does not consider wood as a homogeneous material. The size of the column and type of wood are taken into account. For this example, our column has a depth lesser than or equal to 12 inches. Referencing Table 4D based on the size of the column, a factor of 1.00 is applied. Esta información se puede encontrar en el apartado 4.3.6.2 [1].
Ci – The incising factor considers the preservation treatment applied to the wood to resist decay and avoid fungal growth. Most of the time this involves pressure treatment, but in some cases requires the wood to be incised increasing the surface area for chemical coverage. Para este ejemplo, asumimos que se ha hecho una incisión en la madera. Referencing Table 4.3.8 [1], an overview of the factors by which each member property must be multiplied is shown.
Módulo de elasticidad ajustado
También se deben ajustar los valores de referencia del módulo de elasticidad (E y E min). The adjusted modulus of elasticity (E' and E'min) are determined from Table 4.3.1 [1] and the incising factor Ci is equal to 0.95 from Table 4.3.8 [1].
E' = E ⋅ CM ⋅ Ct ⋅ Ci = 1,140,000.00 psi
E'min = Emin ⋅ CM ⋅ Ct ⋅ Ci = 418,000.00 psi
Column Stability Factor (CP)
The column stability factor (CP) is needed in order to calculate the column's adjusted compressive design value and the compressive design ratio. The following steps will include the necessary equations and values to find CP.
The equation used to calculate CP is Eqn. (3.7-1) referenced in Section 3.7.1.5. The reference compression design value parallel to grain (Fc) is required and calculated below:
F'c = Fc ⋅ CD ⋅ CM ⋅ Ct ⋅ CF ⋅ Ci = 673.40 psi
The next value that needs to be calculated in Eqn. (3.7-1) is the critical buckling design value for compression members (FcE).
The slenderness ratio is calculated as so:
The slenderness ratio is applied to the equation for FcE and the following value is calculated:
FcE = 1342.17 psi
The last variable needed is (c), which is equal to 0.8 for sawn lumber. All of the variables can be applied to Eqn. (3.7-1) and the following value is calculated for CP.
Now, all adjustment factors have been determined from Table 4.3.1 [1]. Therefore, the adjusted compressive design value parallel to grain (F'c) can be calculated.
F'c = Fc ⋅ CD ⋅ CM ⋅ Ct ⋅ CF ⋅ Ci ⋅ CP = 583.602 psi
Razón de tensiones del pilar
El objetivo final de este ejemplo es obtener la razón de tensiones para este pilar simple. Esto va a determinar si el tamaño de la barra es adecuado bajo la carga dada o si se debe optimizar aún más. Calculating the design ratio requires the adjusted compressive design value parallel to the grain about both axes (F'c) and actual compressive stress parallel to the grain (fc). In this case, the cross-section is symmetrical, so F'c is equivalent for both the x- and the y-axis.
The actual compressive stress (fc) is calculated below:
The adjusted compressive design value parallel to the grain (F'c) and the actual compressive stress (fc) are used to compile the design ratio (η) as per Sec. 3.6.3.
Verificación de RFEM 6
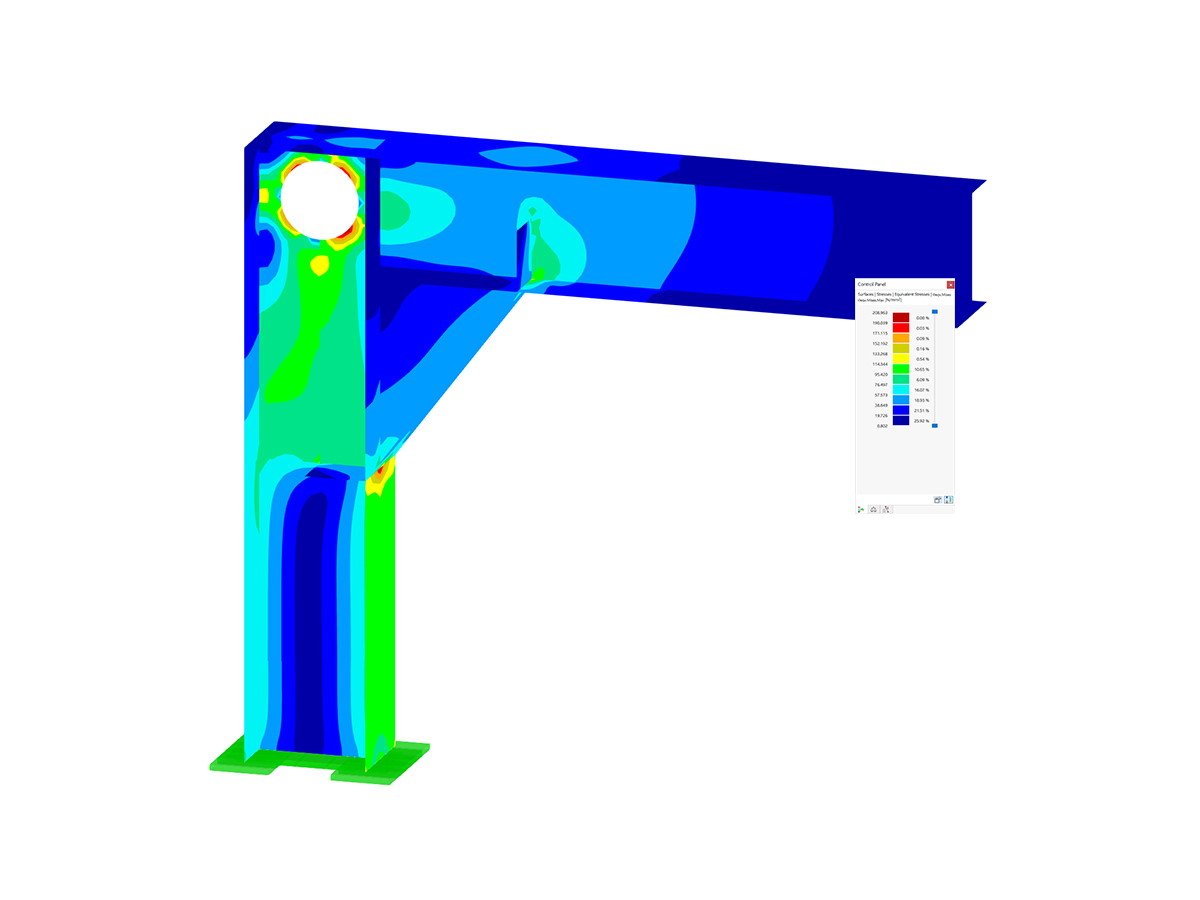
When designing timber per the 2018 NDS standard in RFEM 6, the Timber Design Add-on analyzes and optimizes cross-sections based on loading criteria and member capacity for a single member or a set of members. Esto está disponible para los métodos de cálculo LRFD o ASD. The results between the analytical example and RFEM 6 are compared and verified below.
Editing the Member is where the Design Properties like the Effective Lengths, Service Conditions, Design Configurations and Design Supports can be adjusted for design. The material and cross-section are defined here as well. The moisture service condition is set to Wet and the temperature is equal to or less than 100 degrees Fahrenheit. Lateral-Torsional Buckling is defined according to Table 3.3.3 [1]. The material is set to "User-Defined" and considered "Incised".
Adjusted Compression Design Value Parallel to Grain:
F'c = 1.000
Design Ratio:
η = 1.000
