Back to Videos
Is this page helpful?
634x
003402
2022-04-26

CP 001230 | Sixpack Lift Station in Filzmoos, Austria

A modern 6-seater chairlift called Sixpack Mooslehen opened in Filzmoos, Austria on December 17, 2021, right on time for the start of the ski season. The construction time for the entire project was only six months. The company m3-ZT, an Austrian customer of Dlubal, was responsible for the structural analysis, using the FEA program RFEM.

  • Mia: AI Assistant
  • Latest Videos
Videos
Models to Download
Knowledge Base Articles
Determination of Shear Stresses for Null Elements
In cross‑sections created in SHAPE‑THIN, the openings, such as bolt holes, can be modeled by using the elements with zero thickness. The program provides two options for calculating shear stresses in the area of such null elements.
Editing Member with Variable SHAPE-THIN Cross-Section
In RFEM and RSTAB, you can analyze members with a variable cross-section, which can also consist of freely defined SHAPE-THIN cross-sections. The cross-section properties are interpolated in order to determine the internal forces and deformations.
Modell eines Stahlbetonbalkens, der durch Kriechen und Schwinden beeinflusst wird
This technical article addresses the direct deformation analysis of reinforced concrete beams considering the long-term effects of creep and shrinkage. The direct calculation according to Eurocode 2 (EN 1992-1-1, Section 7.4.3) is explained using a single-span beam. Particular emphasis is placed on tension stiffening, behavior in the cracked state based on the distribution factor (damage parameter), and consideration of shrinkage and creep behavior.
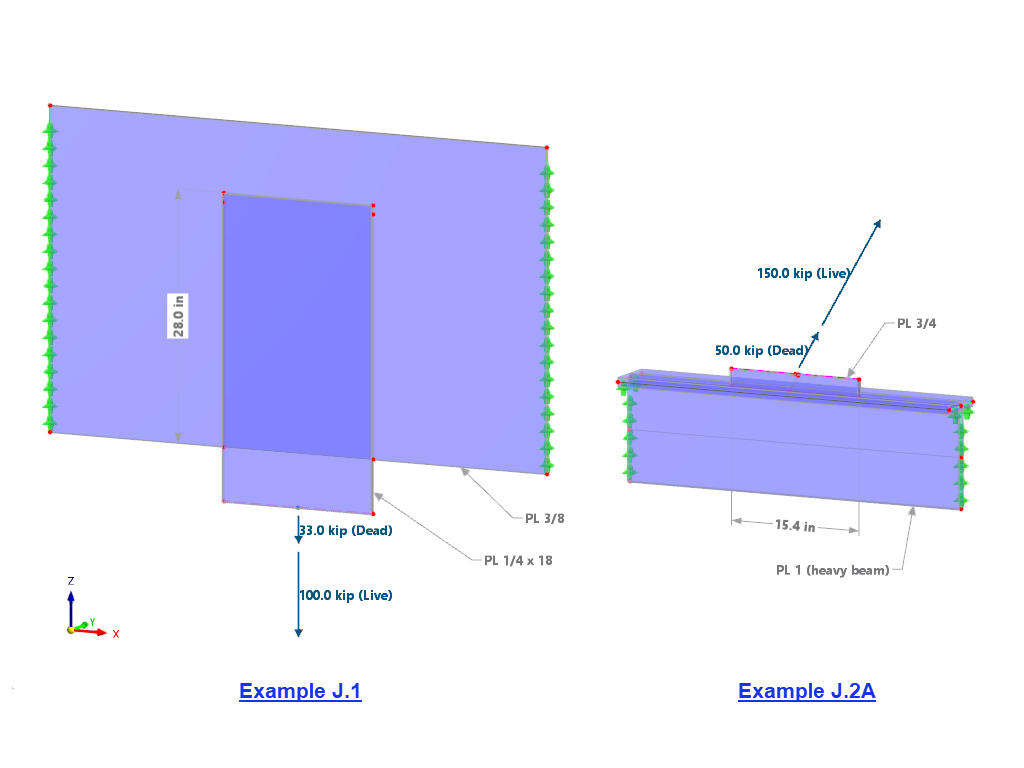
KB 001925 | AISC Fillet Weld Design in RFEM 6
The weld stresses between surfaces can be determined using the Stress-Strain Analysis add-on in RFEM 6. Furthermore, the stress limit determined according to the applicable standard can be input to determine the stress ratio of the weld. This article focuses on the fillet weld design according to AISC 360-22 [1] with two examples from AISC Volume 1: Design Examples [2].
Screenshots
Product Features
Feature 002842 | Sign-Dependent Stress Analysis

In the Stress-Strain Analysis add-on, you can use the option to specify sign-dependent limit stresses by stress component.

Feature 002827 | Stresses Within Members

Get a better understanding of the stress distribution within member cross-sections by using clipping planes.

Feature 002426 | Animation of Deformation

The deformation process of the global deformation components can be represented as a movement sequence.

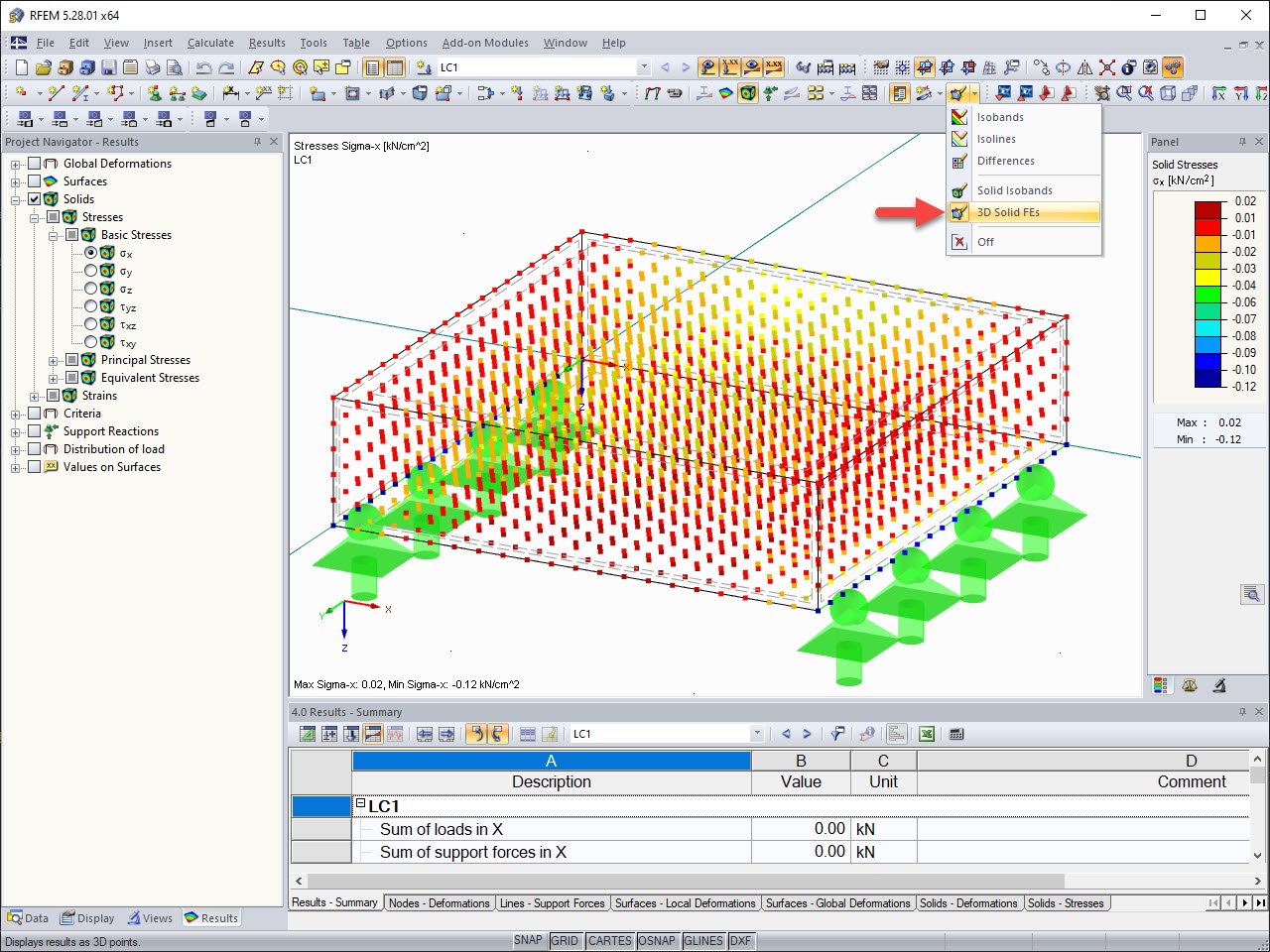
Feature 002423 | Displaying Results in Solids

The results of solid stresses can be displayed as colored 3D points in the finite elements.

Frequently Asked Questions (FAQ)
What is the difference between SHAPE-THIN 9 and SHAPE-THIN 8?
What is the purpose of a fillet weld in SHAPE‑THIN?
How can I display shear stresses on null elements in SHAPE‑THIN?
When calculating a cross-section, I get a message saying that the weld is not connected to two elements. What can I do?
I would like to calculate the results, such as ordinates, static moments, and stresses, at a specific location of a cross-section. What can I do?
How can I calculate stresses in SHAPE‑THIN?
Customer Projects