Answer:
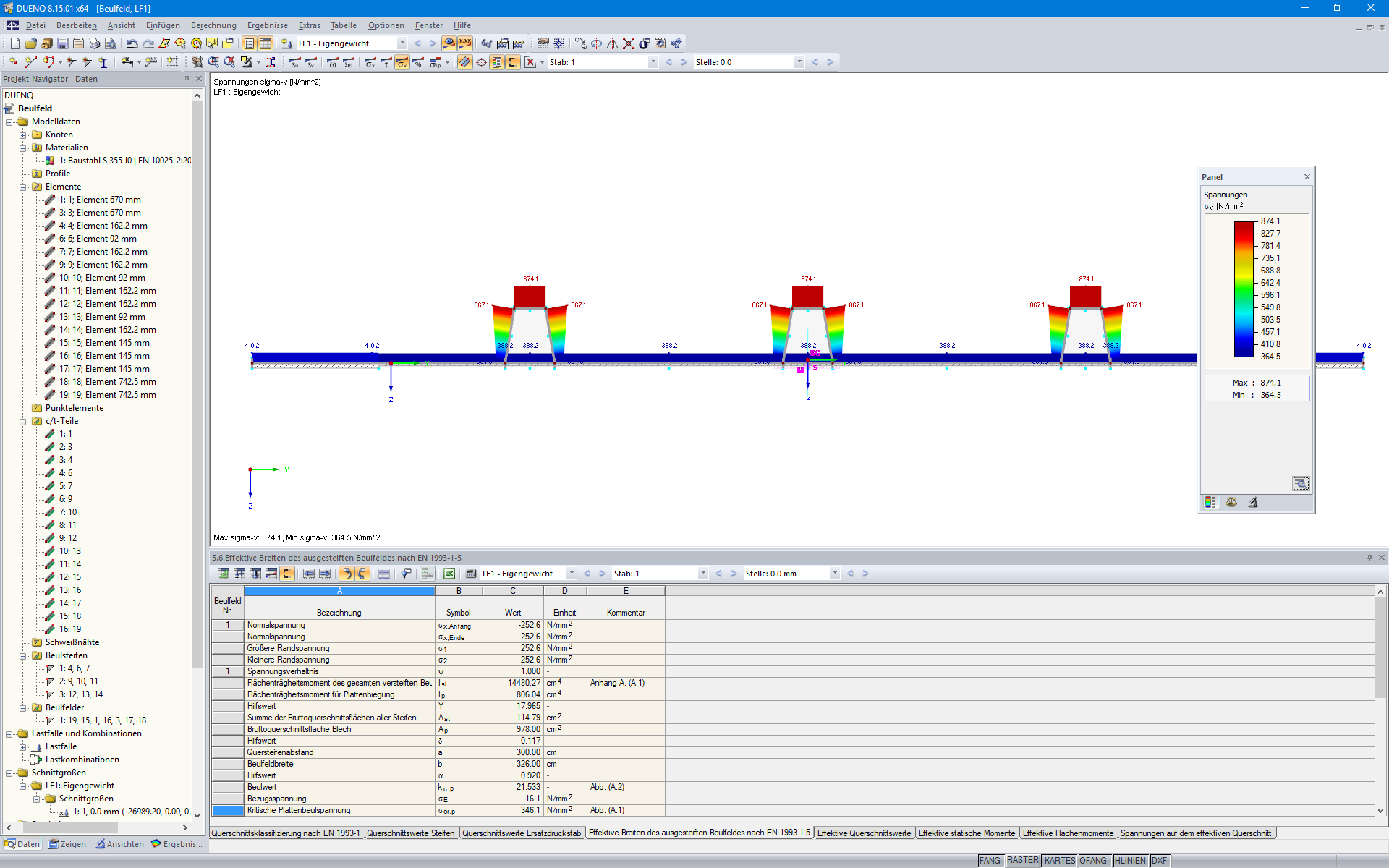
Finite elements with plastic material are divided into 10 layers. Initially, a normal elastic analysis is performed in the first iteration. Then, the stress in each element is calculated according to the set strength hypothesis in each individual layer. If the limit stress in one of the layers is exceeded, the stiffness of this layer is reduced. Based on the reduced stiffnesses of the 10 layers, an overall stiffness is determined for each element. Using this new stiffness, it is possible to start a new iteration of the calculation.
It is iterated as long as the changes are only small.
The total stress is converted into the stresses of the individual layers using the laminate analysis.
This analysis is also applied for the conversion between the stiffnesses of the layers and the total stiffness.
Four different strength hypotheses can be used as the limit stress:
- Shape modification hypothesis (von Mises)
- Maximum shear stress hypothesis (Tresca)
- Failure hypothesis according to Drucker-Prager
- Failure hypothesis according to Mohr-Coloumb
The selection of the failure hypothesis is shown in Image 01.
The von Mises hypothesis is preset, as it is the most frequently used strength hypothesis.
