402x

2020-12-07

VE0019 | Flexión plástica – Momento cargado

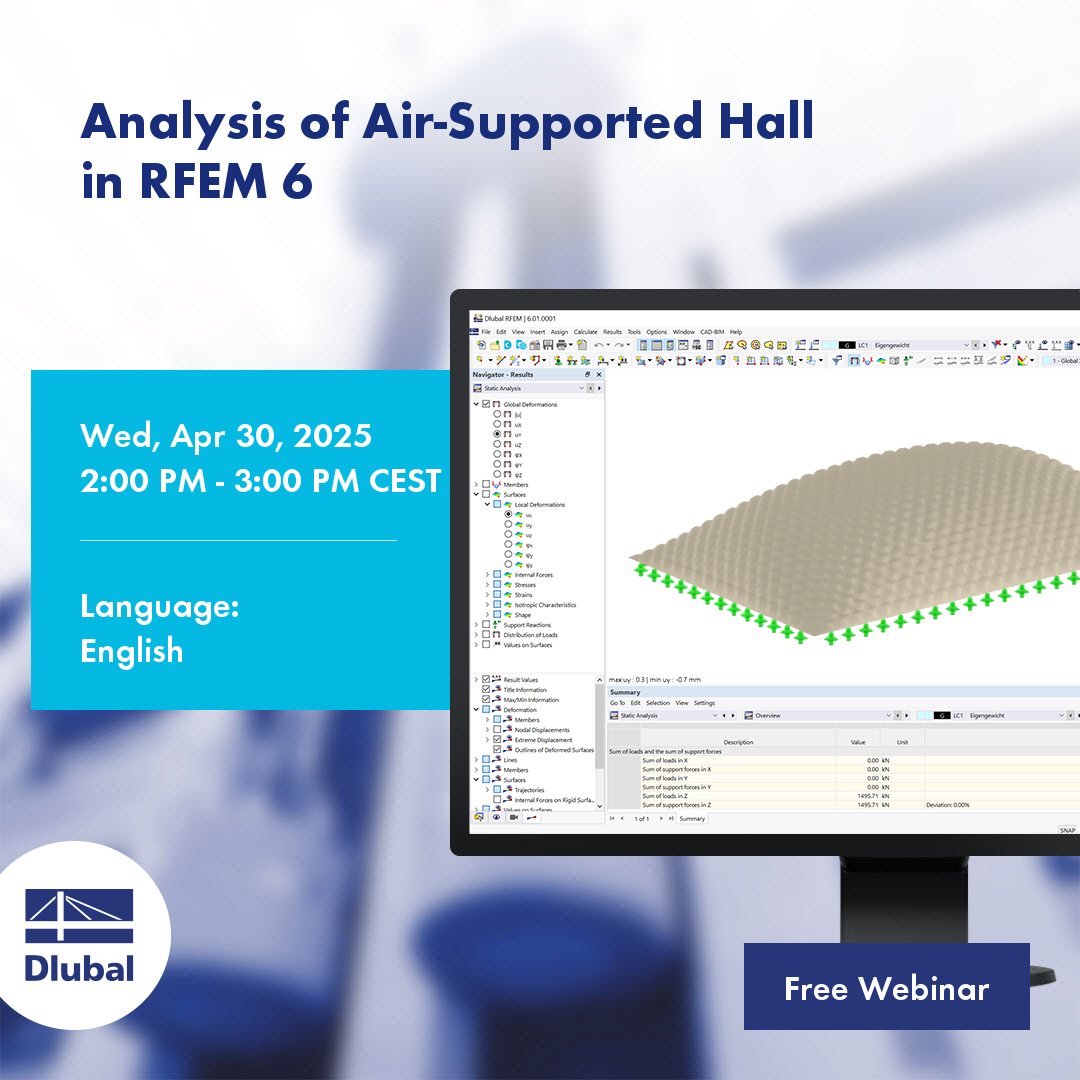
Descripción del trabajo

Un voladizo está completamente fijado en el extremo izquierdo y cargado por un momento flector según el siguiente croquis. The problem is described by the following set of parameters. Small deformations are considered and the self-weight is neglected in this example. Determine the maximum deflection u_z,max.

Material	Elastic-Plastic	Módulo de elasticidad	E	210000.000	MPa
		coeficiente de Poisson	ν	0.000	-
		Módulo de cortante	G	105000.000	MPa
		Plastic Strength	f_y	240.000	MPa
Geometry	Cantilever	perímetro	L	2.000	m
		Ancho	w	0.005	m
		Espesor	t	0.005	m
Load		Momento flector	M	6.000	Nm

Flexión plástica – Momento cargado

Solución analítica

The cantilever is loaded by the bending moment M. The quantities of this load are discussed at first. The moment M_e when the first yield occurs and the ultimate moment M_p when the structure becomes plastic hinge are calculated as follows:

M_{e} = 2 \int_{0}^{t / 2} σ (z) z w d z = 2 \int_{0}^{t / 2} \frac{2 f_{y}}{t} z^{2} w d z = \frac{f_{y} w t^{2}}{6}

Momento elástico

M_{p} = 2 \int_{0}^{t / 2} σ (z) z w d z = 2 \int_{0}^{t / 2} f_{y} z w d z = \frac{f_{y} w t^{2}}{4}

Momento plástico

The bending moment M causes the elastic-plastic state. The cross-section in the elastic-plastic state is divided into the elastic core and the plastic surface, which is described by the parameter z_p according to the following diagram.

Distribución de la tensión de flexión

z_{p} = \frac{f_{y}}{κ (x) E}

Parámetro z_p

The elastic-plastic moment M_ep in the cross-section has to equal to the bending moment M. The curvature κ results from this equality.

κ = \frac{f_{y}}{E} \sqrt{\frac{1}{3 (\frac{t^{2}}{4} - \frac{M}{f_{y} w})}}

curvatura

The total deflection of the structure u_z,max is calculated using the Mohr's integral.

u_{z, \max} = \int_{0}^{L} κ (L - x) d x

Flecha total del voladizo

RFEM Settings

Modeled in RFEM 5.16 and RRFEM 6.01
The element size is l_FE= 0.020 m
Geometrically linear analysis is considered
The number of increments is 5
Shear stiffness of the members is neglected

Resultados

Material Model	Solución analítica	RFEM 5		RFEM 6
Material Model	u_z,max [m]	u_z,max [m]	Ratio [-]	u_z,max [m]	Ratio [-]
Ortótropo plástico 2D	1.180	1.190	1.008	1.190	1.008
Isotropic Plastic 2D/3D, Plate		1.173	0.994	1.173	0.994
Isótropo plástico 1D		1.180	1.000	1.180	1.000
Isotropic Nonlinear Elastic 2D/3D, Plate, Mises		1.190	1.008	1.190	1.008
Isotropic Nonlinear Elastic 2D/3D, Plate, Tresca		1.190	1.008	1.190	1.008
Isótropo plástico 1D		1.180	1.000	1.180	1.000

428x

Ejemplo de verificación 0019 | 1 | Chapa | Ortótropo plástico 2D | Tsai-Wu

Referencias

Lubliner, J. (1990). Teoría de la plasticidad. Nueva York: Macmillan.

;