Analytical Calculation Method
RF-CONCRETE Deflect provides the analytical calculation method according to DIN EN 1992-1-1 [1] 7.4.3. This means that the cracking or contribution of the concrete in the tension zone is determined by means of the distribution coefficient ζ. The structural system can be between state I (uncracked, ζ = 0) and state II (cracked, ζ = 1). In RF-CONCRETE Deflect, a mean stiffness is finally determined with ζ. Based on these values, the deformation is recalculated. Due to the stiffness change, however, no recalculation of the distribution of internal forces will be performed. The method is thus non-iterative. You can find more detailed information about RF-CONCRETE Deflect in Chapter 2.7 of the RF-CONCRETE Surfaces manual [2].
Physical-Nonlinear Calculation Method
RF-CONCRETE NL provides a physical-nonlinear calculation method. The calculation is carried out iteratively. This means that a stiffness is determined based on the loading, which in turn determines the distribution of internal forces. This calculation runs as an iterative process. The iterations run until a convergence criterion is achieved. This is the case if either the stiffness change or the deformation change in relation to the previous iteration step is below the convergence criterion.
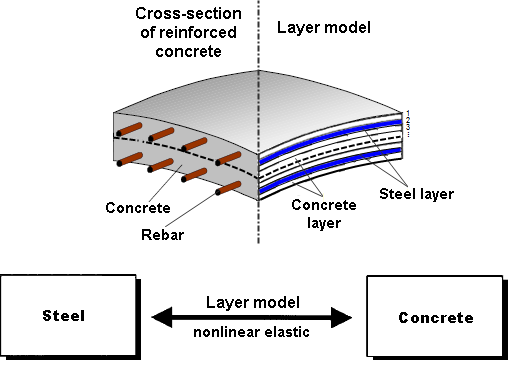
Internally, the FE element is displayed in layers when using the nonlinear calculation method where different materials are assigned to the individual layers (reinforcement and concrete) and the individual concrete layers can have different stiffnesses during the calculation (cracking of the concrete).
You can find detailed information about this topic in the RF-CONCRETE Surfaces manual [2] in Chapter 2.8.
Comparing Features of both Methods
Analytical Calculation Method | RF-CONCRETE Deflect
A very fast calculation method, also suitable for large structures.
Based on the theoretical approach from DIN EN 1992-1-1 [1] 7.4.3, it should only be used for structural components subjected to bending (plates).
Creep is applied to the entire cross-section as a reduction of elastic modulus for concrete.
Due to the shrinkage, an additional distortion is determined, which is added to the deformations.
Physical-Nonlinear Calculation Method | RF-CONCRETE NL
A very precise and all-purpose method, not limited solely to structural components mainly subjected to bending (for example, it can also be used for deep beams).
Creep is applied to the entire cross-section in the compression zone as a reduction of elastic modulus for concrete.
Shrinkage is considered internally on the load side as strain, but in this context, failure due to shrinkage strain is also possible.
This calculation is much more complex than the analytical method and is, therefore, considerably more computationally intensive.
Application Areas of These Methods
Four criteria are essential in deciding which method should be used for a particular situation.
1. Loading Situation
For structures where individual structural components with a different loading situation act together, the nonlinear method should be used because it is not limited to structural components that are subjected to bending. One example is a cantilevered floor.
Due to the load situation, a structure that has mainly wall stresses should also be analyzed with the physical-nonlinear method from RF-CONCRETE NL. One example of this is a deep beam.
2. Structure Size
For large structures, we recommend attempting to avoid the physical-nonlinear method, because it requires a great deal of computing time.
3. Required Accuracy
If the deformation design according to DIN EN 1992-1-1 [1] 7.4.1 (4) has to be carried out for the visual appearance, the simpler analytical method may be sufficient, and no accurate method has to be selected unnecessarily for the design.
If the deformation design according to DIN EN 1992-1-1 [1] 7.4.1 (5) has to be carried out to avoid damaging adjacent structural components or if spacers have to be dimensioned, it is worth taking a closer look in connection with the design purpose. Under certain circumstances, it may be important not only that the determined deformation value is smaller than the required limit value, but that the deformation is also determined as precisely as possible.
4. Considering Special Effects
Shrinkage is particularly important here. While additional curvature due to shrinkage is determined when using the analytical method in RF-CONCRETE Deflect, the shrinkage can be considered more precisely when using the physical-nonlinear method. In RF-CONCRETE NL, the shrinkage is taken into account internally on the load side as elongation and can lead to additional curvature as well as additional effects due to restraint in the structure. As a result of the effects of restraint, shrinkage may in turn lead to cracking of the concrete. In this context, it is important to display the supports as precisely as possible, in particular the horizontal supports.
Furthermore, when using the physical-nonlinear calculation, it is possible to determine an additional value for the crack width direction. By layering the element, the crack depth can be determined and displayed.